안녕하세요. 지난 포스팅의 미적분학 - 매개변수와 미적분학에서는 매개변수 함수로 주어진 함수의 기울기, 영역의 넓이, 곡선의 길이, 곡면의 겉넓이를 구하는 방법에 대해서 알아보았습니다. 오늘은 매개변수화(parameterization)의 가장 대표적인 예인 극좌표(Polar Coordinate)에 대해서 알아보도록 하겠습니다.
일반적으로 저희가 사용하는 직교좌표계는 "르네 데카르트"라는 프랑스 철학자이자 수학자가 개발하였습니다. 직교좌표계는 카르테시안 좌표계(Cartesian Coordinate System)이라고도 불립니다. 일상생활의 많은 부분에서는 직교좌표계를 이용하면 쉽게 물체의 위치를 알 수 있습니다. 하지만, 모다 복잡한 움직임을 표현하기에는 어려운데요, 이를 보완하고자 뉴턴이 만든 좌표계가 극좌표계입니다.
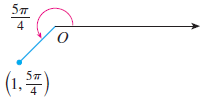
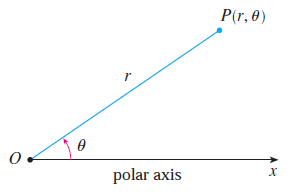
극좌표계는 위의 그림과 같이 2개의 정보를 이용해서 물체의 위치를 정의합니다. 물체 $P$의 위치를 표현하고 싶다면 일단, 원점 $O$를 정의하고 원점으로부터 물체까지의 거리 $r$과 극축(Polar Axis)을 기준으로 물체 $P$가 반시계방향으로 몇 도 $\theta$에 위치하는 지를 이용합니다. 따라서, 물체 $P$는 $(r, \theta)$로 표현할 수 있습니다. 예를 들어, $(1, 5\pi / 4)$는 원점으로부터 거리는 1이고 반시계방향으로 각도가 $\frac{5\pi}{4}$인 점을 의미합니다.
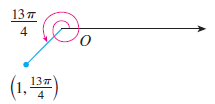
여기서 극좌표의 중요한 성질을 볼 수 있습니다. $2\pi$는 360도로 제자리이기 때문에 $(r, \theta) = (r, \theta + 2\pi)$입니다. 근데, $4\pi$ 역시 720도이기 때문에 제자리입니다. 따라서, $(r, \theta) = (r, \theta + 4\pi)$이죠. 이를 임의의 정수 $n$에 대해서 일반화하면 아래와 같습니다.
$$(r, \theta) = (r, \theta + 2n\pi)$$
마지막으로 직교좌표계와 극좌표계를 서로 변환할 수 있는 방법에 대해서 알아보도록 하겠습니다. 기본적으로 직교좌표계에서 극좌표계로 바꾸는 방법은 극좌표계의 정의를 보면 됩니다. $r$은 원점으로부터 점까지의 거리이고 $\theta$는 극축의 반시계방향으로의 각도를 의미합니다. 따라서, $r = \sqrt{x^{2} + y^{2}}, \theta = \arctan(\frac{y}{x})$로 정의하면 됩니다. 그렇다면, 극좌표계에서 직교좌표계로는 어떻게 변환할 수 있을까요? 이 역시 간단합니다. $x = r\cos(\theta), y = r\sin(\theta)$로 정의하면 됩니다. 정리하면 아래와 같습니다.
- 직교좌표계 $\rightarrow$ 극좌표계 : $(r, \theta) = (\sqrt{x^{2} + y^{2}}, \arctan(\frac{y}{x}))$
- 극좌표계 $\rightarrow$ 직교좌표계 : $(x, y) = (r\cos(\theta), r\sin(\theta))$
예제1. 극좌표계 $(2, \frac{2\pi}{3})$를 직교좌표계로 변환하라.
$$(x, y) = (2\cos(\frac{2\pi}{3}), 2\sin(\frac{2\pi}{3})) = (2 \cdot \frac{1}{2}, 2 \cdot \frac{\sqrt{3}}{2}) = (1, \sqrt{3})$$
예제2. 직교좌표계 $(1, -1)$를 극좌표계로 변환하라.
$$(r, \theta) = (\sqrt{(-1)^{2} + 1^{2}}, \arctan(-1)) = (\sqrt{2}, \frac{7\pi}{4})$$
연습문제1. 주어진 극좌표계의 점을 직교좌표계로 변환하라.
(a). $(2, \frac{\pi}{3})$
(b). $(1, \frac{-4\pi}{3})$
(c). $(-1, \frac{\pi}{2})$
(d). $(1, \frac{7\pi}{4})$
(e). $(-3, \frac{\pi}{6})$
(a). $(2, \frac{\pi}{3})$
$$\begin{cases} x &= r\cos(\theta) = 2 \cdot \cos(\frac{\pi}{3}) = 1 \cdot \frac{1}{2} = 1 \\ y &= r\sin(\theta) = 2 \cdot \sin(\frac{\pi}{3}) = 2 \cdot \frac{\sqrt{3}}{2} = \sqrt{3} \end{cases}$$
따라서, $(2, \frac{\pi}{3})$의 직교좌표계는 $(1, \sqrt{3})$이다.
(b). $(1, \frac{-4\pi}{3})$
$$\begin{cases} x &= r\cos(\theta) = 1 \cdot \cos(-\frac{4\pi}{3}) = 1 \cdot (-\frac{1}{2}) = -\frac{1}{2} \\ y &= r\sin(\theta) = 1 \cdot \sin(\frac{-4\pi}{3}) = 1 \cdot \frac{\sqrt{3}}{2} = \frac{\sqrt{3}}{2} \end{cases}$$
따라서, $(1, \frac{-4\pi}{3})$의 직교좌표계는 $(-\frac{1}{2}, \frac{\sqrt{3}}{2})$이다.
(c). $(-1, \frac{\pi}{2})$
$$\begin{cases} x &= r\cos(\theta) = (-1) \cdot \cos(\frac{\pi}{2}) = (-1) \cdot 0 = 0 \\ y &= r\sin(\theta) = (-1) \cdot \sin(\frac{\pi}{2}) = (-1) \cdot 1 = -1 \end{cases}$$
따라서, $(-1, \frac{\pi}{2})$의 직교좌표계는 $(0, -1)$이다.
(d). $(1, \frac{7\pi}{4})$
$$\begin{cases} x &= r\cos(\theta) = 1 \cdot \cos(\frac{7\pi}{4}) = 1 \cdot (-\frac{\sqrt{2}}{2}) = -\frac{\sqrt{2}}{2} \\ y &= r\sin(\theta) = 1 \cdot \sin(\frac{7\pi}{4}) = 1 \cdot \frac{\sqrt{2}}{2} = \frac{\sqrt{2}}{2} \end{cases}$$
따라서, $(1, \frac{7\pi}{4})$의 직교좌표계는 $(-\frac{\sqrt{2}}{2}, \frac{\sqrt{2}}{2})$이다.
(e). $(-3, \frac{\pi}{6})$
$$\begin{cases} x &= r\cos(\theta) = (-3) \cdot \cos(\frac{\pi}{6}) = (-3) \cdot \frac{\sqrt{3}}{2} = -\frac{3\sqrt{3}}{2} \\ y &= r\sin(\theta) = (-3) \cdot \sin(\frac{\pi}{6}) = (-3) \cdot \frac{1}{2} = -\frac{3}{2} \end{cases}$$
따라서, $(-3, \frac{\pi}{6})$의 직교좌표계는 $(-\frac{3\sqrt{3}}{2}, -\frac{3}{2})$이다.
연습문제2. 주어진 극좌표계의 점을 직교좌표계로 변환하라.
(a). $(1, \pi)$
(b). $(2, -\frac{2\pi}{3})$
(c). $(-2, \frac{3\pi}{4})$
(d). $(-\sqrt{2}, \frac{5\pi}{4})$
(e). $(1, \frac{5\pi}{2})$
(f). $(2, -\frac{7\pi}{6})$
(a). $(1, \pi)$
$$\begin{cases} x &= r\cos(\theta) = 1 \cdot \cos(\pi) = 1 \cdot (-1) = -1 \\ y &= r\sin(\theta) = 1 \cdot \sin(\pi) = 1 \cdot 0 = 0 \end{cases}$$
따라서, $(1, \pi)$의 직교좌표계는 $(-1, 0)$이다.
(b). $(2, -\frac{2\pi}{3})$
$$\begin{cases} x &= r\cos(\theta) = 2 \cdot \cos(-\frac{2\pi}{3}) = 2 \cdot (-\frac{1}{2}) = -1 \\ y &= r\sin(\theta) = 2 \cdot \sin(-\frac{2\pi}{3}) = 2 \cdot (-\frac{\sqrt{3}}{2}) = -\sqrt{3} \end{cases}$$
따라서, $(2, -\frac{2\pi}{3})$의 직교좌표계는 $(-1, -\sqrt{3})$이다.
(c). $(-2, \frac{3\pi}{4})$
$$\begin{cases} x &= r\cos(\theta) = (-2) \cdot \cos(\frac{3\pi}{4}) = (-2) \cdot (-\frac{\sqrt{2}}{2}) = \sqrt{2} \\ y &= r\sin(\theta) = (-2) \cdot \sin(\frac{3\pi}{4}) = (-2) \cdot \frac{\sqrt{2}}{2} = -\sqrt{2} \end{cases}$$
따라서, $(-2, \frac{3\pi}{4})$의 직교좌표계는 $(\sqrt{2}, -\sqrt{2})$이다.
(d). $(-\sqrt{2}, \frac{5\pi}{4})$
$$\begin{cases} x &= r\cos(\theta) = (-\sqrt{2}) \cdot \cos(\frac{5\pi}{4}) = (-\sqrt{2}) \cdot (-\frac{\sqrt{2}}{2}) = 1 \\ y &= r\sin(\theta) = (-\sqrt{2}) \cdot \sin(\frac{5\pi}{4}) = (-\sqrt{2}) \cdot (-\frac{\sqrt{2}}{2}) = 1 \end{cases}$$
따라서, $(-\sqrt{2}, \frac{5\pi}{4})$의 직교좌표계는 $(1, 1)$이다.
(e). $(1, \frac{5\pi}{2})$
$$\begin{cases} x &= r\cos(\theta) = 1 \cdot \cos(\frac{5\pi}{2}) = 1 \cdot 0 = 0 \\ y &= r\sin(\theta) = 1 \cdot \sin(\frac{5\pi}{2}) = 1 \cdot 1 = 1 \end{cases}$$
따라서, $(1, \frac{5\pi}{2})$의 직교좌표계는 $(0, 1)$이다.
(f). $(2, -\frac{7\pi}{6})$
$$\begin{cases} x &= r\cos(\theta) = 2 \cdot \cos(-\frac{7\pi}{6}) = 2 \cdot (-\frac{\sqrt{3}}{2}) = -\sqrt{3} \\ y &= r\sin(\theta) = 2 \cdot \sin(-\frac{7\pi}{6}) = 2 \cdot \frac{1}{2} = 1 \end{cases}$$
따라서, $(2, -\frac{7\pi}{6})$의 직교좌표계는 $(-\sqrt{3}, 1)$이다.
연습문제3. 극좌표계에서 정의된 두 점 $(2, \frac{\pi}{3})$와 $(4, \frac{2\pi}{3})$ 사이의 거리를 구하여라.
먼저, 두 극좌표계에서 정의된 점을 직교좌표계로 변환한다.
1). $(2, \frac{\pi}{3})$
$$\begin{cases} x &= r\cos(\theta) = 2 \cdot \cos(\frac{\pi}{3}) = 2 \cdot \frac{1}{2} = 1 \\ y &= r\sin(\theta) = 2 \cdot \sin(\frac{\pi}{3}) = 2 \cdot \frac{\sqrt{3}}{2} = \sqrt{3} \end{cases}$$
따라서, $(2, \frac{\pi}{3})$의 직교좌표계는 $(1, \sqrt{3})$이다.
2). $(4, \frac{2\pi}{3})$
$$\begin{cases} x &= r\cos(\theta) = 4 \cdot \cos(\frac{2\pi}{3}) = 4 \cdot (-\frac{1}{2}) = -2 \\ y &= r\sin(\theta) = 4 \cdot \sin(\frac{2\pi}{3}) = 4 \cdot \frac{\sqrt{3}}{2} = 2\sqrt{3} \end{cases}$$
따라서, $(4, \frac{2\pi}{3})$의 직교좌표계는 $(-2, 2\sqrt{3})$이다.
1)과 2)의 결과를 이용하여 두 점 사이의 거리를 구하는 공식을 사용하면 된다.
$$D = \sqrt{(1 + 2)^{2} + (\sqrt{3} - 2\sqrt{3})^{2}} = \sqrt{9 + 3} = \sqrt{12} = 2\sqrt{3}$$
연습문제4. 극좌표계에서 정의된 두 점 $(r_{1}, \theta_{1})$와 $(r_{2}, \theta_{2})$ 사이의 거리를 구하여라.
극좌표계에서 직교좌표계로 변환하면 각 점은 $(r_{1}\cos(\theta_{1}), r_{1}\sin(\theta_{1}))$과 $(r_{2}\cos(\theta_{2}), r_{2}\sin(\theta_{2}))$이다. 따라서, 두 점 사이의 거리를 구하는 공식을 이용하면 다음과 같다.
$$\begin{align*} d^{2} &= (r_{1}\cos(\theta_{1}) - r_{2}\cos(\theta_{2}))^{2} + (r_{1}\sin(\theta_{1}) - r_{2}\sin(\theta_{2}))^{2} \\ &= (r^{2}_{1}\cos^{2} (\theta_{1}) - 2r_{1}r_{2}\cos(\theta_{1})\cos(\theta_{2}) + r^{2}_{2}\cos^{2} (\theta_{2}) ) \\ &+ (r^{2}_{1}\sin^{2} (\theta_{1}) - 2r_{1}r_{2}\sin(\theta_{1})\sin(\theta_{2}) + r^{2}_{2}\sin^{2} (\theta_{2})) \\ &= r^{2}_{1} (\sin^{2} (\theta_{1}) + \cos^{2} (\theta_{1})) - 2r_{1}r_{2} (\sin(\theta_{1})\sin(\theta_{2}) + \cos(\theta_{1})\cos(\theta_{2})) + r^{2}_{2} (\sin^{2} (\theta_{2}) + \cos^{2} (\theta_{2})) \\ &= r^{2}_{1} + r^{2}_{2} - 2r_{1}r_{2} \cos(\theta_{1} - \theta_{2}) \end{align*}$$
최종적으로 두 점 사이의 거리는 $d = \sqrt{r^{2}_{1} + r^{2}_{2} - 2r_{1}r_{2}\cos(\theta_{1} - \theta_{2})}$이다.
연습문제5. 극좌표계에서 정의된 곡선들을 직교좌표계로 변환하라.
(a). $r = 2$
(b). $r\cos(\theta) = 1$
(c). $r = 3\sin(\theta)$
(d). $r = 2\sin(\theta) + 2\cos(\theta)$
(e). $r = \csc(\theta)$
(f). $r = \tan(\theta) \sec(\theta)$
(a). $r = 2$
$$r = 2 \Rightarrow x^{2} + y^{2} = 4$$
(b). $r\cos(\theta) = 1$
$$r\cos(\theta) = 1 \Rightarrow x = 1$$
(c). $r = 3\sin(\theta)$
$$r = 3\sin(\theta) \Rightarrow r^{2} = 3r\sin(\theta) \Rightarrow x^{2} + y^{2} = 3y \Rightarrow x^{2} + (y - \frac{3}{2})^{2} = \frac{9}{4}$$
(d). $r = 2\sin(\theta) + 2\cos(\theta)$
$$r = 2\sin(\theta) + 2\cos(\theta) \Rightarrow r^{2} = 2r\sin(\theta) + 2r\cos(\theta) \Rightarrow x^{2} + y^{2} = 2y + 2x \Rightarrow (x - 1)^{2} + (y - 1)^{2} = 2$$
(e). $r = \csc(\theta)$
$$r = \csc(\theta) \Rightarrow r\sin(\theta) = 1 \Rightarrow y = 1$$
(f). $r = \tan(\theta) \sec(\theta)$
$$r = \tan(\theta) \sec(\theta) = \frac{\sin(\theta)}{\cos^{2} (\theta)} \Rightarrow r^{2}\cos^{2} (\theta) = r\sin(\theta) \Rightarrow x^{2} = y$$
연습문제6. 직교좌표계에서 정의된 곡선들을 극좌표계로 변환하라.
(a). $x = 2$
(b). $x^{2} + y^{2} = 9$
(c). $x = -y^{2}$
(d). $x + y = 9$
(e). $x^{2} + y^{2} = 2cx$
(f). $xy = 4$
(a). $x = 2$
$$x = 2 \Rightarrow r\cos(\theta) = 2$$
(b). $x^{2} + y^{2} = 9$
$$x^{2} + y^{2} = 9 \Rightarrow r^{2} = 9 \Rightarrow r = 3$$
(c). $x = -y^{2}$
$$x = -y^{2} \Rightarrow r \cos(\theta) = -r^{2}\sin(\theta) \Rightarrow r\tan(\theta) = -1$$
(d). $x + y = 9$
$$x + y = 9 \Rightarrow r \cos(\theta) + r\sin(\theta) = r(\cos(\theta) + \sin(\theta)) = 9$$
(e). $x^{2} + y^{2} = 2cx$
$$x^{2} + y^{2} = 2cx \Rightarrow r^{2} = 2rc\cos(\theta) \Rightarrow r = 2c\cos(\theta)$$
(f). $xy = 4$
$$xy = 4 \Rightarrow r\cos(\theta) r\sin(\theta) = r^{2}\cos(\theta)\sin(\theta) = 4 \Rightarrow r^{2}\sin(2\theta) = 8$$
참고자료 및 그림출처
Calculus(J. Stewart)
'수학 > 미적분학' 카테고리의 다른 글
| 미적분학 - 극좌표계 미분과 적분 (0) | 2022.04.10 |
|---|---|
| 미적분학 - 극좌표계 곡선 (0) | 2022.04.09 |
| 미적분학 - 매개변수와 미적분학 (0) | 2022.04.06 |
| 미적분학 - 매개변수 함수 (0) | 2022.04.02 |
| 미적분학 - 일 (0) | 2022.03.30 |
안녕하세요. 지난 포스팅의 미적분학 - 매개변수와 미적분학에서는 매개변수 함수로 주어진 함수의 기울기, 영역의 넓이, 곡선의 길이, 곡면의 겉넓이를 구하는 방법에 대해서 알아보았습니다. 오늘은 매개변수화(parameterization)의 가장 대표적인 예인 극좌표(Polar Coordinate)에 대해서 알아보도록 하겠습니다.

일반적으로 저희가 사용하는 직교좌표계는 "르네 데카르트"라는 프랑스 철학자이자 수학자가 개발하였습니다. 직교좌표계는 카르테시안 좌표계(Cartesian Coordinate System)이라고도 불립니다. 일상생활의 많은 부분에서는 직교좌표계를 이용하면 쉽게 물체의 위치를 알 수 있습니다. 하지만, 모다 복잡한 움직임을 표현하기에는 어려운데요, 이를 보완하고자 뉴턴이 만든 좌표계가 극좌표계입니다.
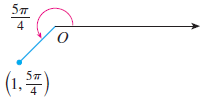
극좌표계는 위의 그림과 같이 2개의 정보를 이용해서 물체의 위치를 정의합니다. 물체 $P$의 위치를 표현하고 싶다면 일단, 원점 $O$를 정의하고 원점으로부터 물체까지의 거리 $r$과 극축(Polar Axis)을 기준으로 물체 $P$가 반시계방향으로 몇 도 $\theta$에 위치하는 지를 이용합니다. 따라서, 물체 $P$는 $(r, \theta)$로 표현할 수 있습니다. 예를 들어, $(1, 5\pi / 4)$는 원점으로부터 거리는 1이고 반시계방향으로 각도가 $\frac{5\pi}{4}$인 점을 의미합니다.
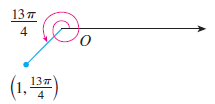
여기서 극좌표의 중요한 성질을 볼 수 있습니다. $2\pi$는 360도로 제자리이기 때문에 $(r, \theta) = (r, \theta + 2\pi)$입니다. 근데, $4\pi$ 역시 720도이기 때문에 제자리입니다. 따라서, $(r, \theta) = (r, \theta + 4\pi)$이죠. 이를 임의의 정수 $n$에 대해서 일반화하면 아래와 같습니다.
$$(r, \theta) = (r, \theta + 2n\pi)$$
마지막으로 직교좌표계와 극좌표계를 서로 변환할 수 있는 방법에 대해서 알아보도록 하겠습니다. 기본적으로 직교좌표계에서 극좌표계로 바꾸는 방법은 극좌표계의 정의를 보면 됩니다. $r$은 원점으로부터 점까지의 거리이고 $\theta$는 극축의 반시계방향으로의 각도를 의미합니다. 따라서, $r = \sqrt{x^{2} + y^{2}}, \theta = \arctan(\frac{y}{x})$로 정의하면 됩니다. 그렇다면, 극좌표계에서 직교좌표계로는 어떻게 변환할 수 있을까요? 이 역시 간단합니다. $x = r\cos(\theta), y = r\sin(\theta)$로 정의하면 됩니다. 정리하면 아래와 같습니다.
- 직교좌표계 $\rightarrow$ 극좌표계 : $(r, \theta) = (\sqrt{x^{2} + y^{2}}, \arctan(\frac{y}{x}))$
- 극좌표계 $\rightarrow$ 직교좌표계 : $(x, y) = (r\cos(\theta), r\sin(\theta))$
예제1. 극좌표계 $(2, \frac{2\pi}{3})$를 직교좌표계로 변환하라.
$$(x, y) = (2\cos(\frac{2\pi}{3}), 2\sin(\frac{2\pi}{3})) = (2 \cdot \frac{1}{2}, 2 \cdot \frac{\sqrt{3}}{2}) = (1, \sqrt{3})$$
예제2. 직교좌표계 $(1, -1)$를 극좌표계로 변환하라.
$$(r, \theta) = (\sqrt{(-1)^{2} + 1^{2}}, \arctan(-1)) = (\sqrt{2}, \frac{7\pi}{4})$$
연습문제1. 주어진 극좌표계의 점을 직교좌표계로 변환하라.
(a). $(2, \frac{\pi}{3})$
(b). $(1, \frac{-4\pi}{3})$
(c). $(-1, \frac{\pi}{2})$
(d). $(1, \frac{7\pi}{4})$
(e). $(-3, \frac{\pi}{6})$
(a). $(2, \frac{\pi}{3})$
$$\begin{cases} x &= r\cos(\theta) = 2 \cdot \cos(\frac{\pi}{3}) = 1 \cdot \frac{1}{2} = 1 \\ y &= r\sin(\theta) = 2 \cdot \sin(\frac{\pi}{3}) = 2 \cdot \frac{\sqrt{3}}{2} = \sqrt{3} \end{cases}$$
따라서, $(2, \frac{\pi}{3})$의 직교좌표계는 $(1, \sqrt{3})$이다.
(b). $(1, \frac{-4\pi}{3})$
$$\begin{cases} x &= r\cos(\theta) = 1 \cdot \cos(-\frac{4\pi}{3}) = 1 \cdot (-\frac{1}{2}) = -\frac{1}{2} \\ y &= r\sin(\theta) = 1 \cdot \sin(\frac{-4\pi}{3}) = 1 \cdot \frac{\sqrt{3}}{2} = \frac{\sqrt{3}}{2} \end{cases}$$
따라서, $(1, \frac{-4\pi}{3})$의 직교좌표계는 $(-\frac{1}{2}, \frac{\sqrt{3}}{2})$이다.
(c). $(-1, \frac{\pi}{2})$
$$\begin{cases} x &= r\cos(\theta) = (-1) \cdot \cos(\frac{\pi}{2}) = (-1) \cdot 0 = 0 \\ y &= r\sin(\theta) = (-1) \cdot \sin(\frac{\pi}{2}) = (-1) \cdot 1 = -1 \end{cases}$$
따라서, $(-1, \frac{\pi}{2})$의 직교좌표계는 $(0, -1)$이다.
(d). $(1, \frac{7\pi}{4})$
$$\begin{cases} x &= r\cos(\theta) = 1 \cdot \cos(\frac{7\pi}{4}) = 1 \cdot (-\frac{\sqrt{2}}{2}) = -\frac{\sqrt{2}}{2} \\ y &= r\sin(\theta) = 1 \cdot \sin(\frac{7\pi}{4}) = 1 \cdot \frac{\sqrt{2}}{2} = \frac{\sqrt{2}}{2} \end{cases}$$
따라서, $(1, \frac{7\pi}{4})$의 직교좌표계는 $(-\frac{\sqrt{2}}{2}, \frac{\sqrt{2}}{2})$이다.
(e). $(-3, \frac{\pi}{6})$
$$\begin{cases} x &= r\cos(\theta) = (-3) \cdot \cos(\frac{\pi}{6}) = (-3) \cdot \frac{\sqrt{3}}{2} = -\frac{3\sqrt{3}}{2} \\ y &= r\sin(\theta) = (-3) \cdot \sin(\frac{\pi}{6}) = (-3) \cdot \frac{1}{2} = -\frac{3}{2} \end{cases}$$
따라서, $(-3, \frac{\pi}{6})$의 직교좌표계는 $(-\frac{3\sqrt{3}}{2}, -\frac{3}{2})$이다.
연습문제2. 주어진 극좌표계의 점을 직교좌표계로 변환하라.
(a). $(1, \pi)$
(b). $(2, -\frac{2\pi}{3})$
(c). $(-2, \frac{3\pi}{4})$
(d). $(-\sqrt{2}, \frac{5\pi}{4})$
(e). $(1, \frac{5\pi}{2})$
(f). $(2, -\frac{7\pi}{6})$
(a). $(1, \pi)$
$$\begin{cases} x &= r\cos(\theta) = 1 \cdot \cos(\pi) = 1 \cdot (-1) = -1 \\ y &= r\sin(\theta) = 1 \cdot \sin(\pi) = 1 \cdot 0 = 0 \end{cases}$$
따라서, $(1, \pi)$의 직교좌표계는 $(-1, 0)$이다.
(b). $(2, -\frac{2\pi}{3})$
$$\begin{cases} x &= r\cos(\theta) = 2 \cdot \cos(-\frac{2\pi}{3}) = 2 \cdot (-\frac{1}{2}) = -1 \\ y &= r\sin(\theta) = 2 \cdot \sin(-\frac{2\pi}{3}) = 2 \cdot (-\frac{\sqrt{3}}{2}) = -\sqrt{3} \end{cases}$$
따라서, $(2, -\frac{2\pi}{3})$의 직교좌표계는 $(-1, -\sqrt{3})$이다.
(c). $(-2, \frac{3\pi}{4})$
$$\begin{cases} x &= r\cos(\theta) = (-2) \cdot \cos(\frac{3\pi}{4}) = (-2) \cdot (-\frac{\sqrt{2}}{2}) = \sqrt{2} \\ y &= r\sin(\theta) = (-2) \cdot \sin(\frac{3\pi}{4}) = (-2) \cdot \frac{\sqrt{2}}{2} = -\sqrt{2} \end{cases}$$
따라서, $(-2, \frac{3\pi}{4})$의 직교좌표계는 $(\sqrt{2}, -\sqrt{2})$이다.
(d). $(-\sqrt{2}, \frac{5\pi}{4})$
$$\begin{cases} x &= r\cos(\theta) = (-\sqrt{2}) \cdot \cos(\frac{5\pi}{4}) = (-\sqrt{2}) \cdot (-\frac{\sqrt{2}}{2}) = 1 \\ y &= r\sin(\theta) = (-\sqrt{2}) \cdot \sin(\frac{5\pi}{4}) = (-\sqrt{2}) \cdot (-\frac{\sqrt{2}}{2}) = 1 \end{cases}$$
따라서, $(-\sqrt{2}, \frac{5\pi}{4})$의 직교좌표계는 $(1, 1)$이다.
(e). $(1, \frac{5\pi}{2})$
$$\begin{cases} x &= r\cos(\theta) = 1 \cdot \cos(\frac{5\pi}{2}) = 1 \cdot 0 = 0 \\ y &= r\sin(\theta) = 1 \cdot \sin(\frac{5\pi}{2}) = 1 \cdot 1 = 1 \end{cases}$$
따라서, $(1, \frac{5\pi}{2})$의 직교좌표계는 $(0, 1)$이다.
(f). $(2, -\frac{7\pi}{6})$
$$\begin{cases} x &= r\cos(\theta) = 2 \cdot \cos(-\frac{7\pi}{6}) = 2 \cdot (-\frac{\sqrt{3}}{2}) = -\sqrt{3} \\ y &= r\sin(\theta) = 2 \cdot \sin(-\frac{7\pi}{6}) = 2 \cdot \frac{1}{2} = 1 \end{cases}$$
따라서, $(2, -\frac{7\pi}{6})$의 직교좌표계는 $(-\sqrt{3}, 1)$이다.
연습문제3. 극좌표계에서 정의된 두 점 $(2, \frac{\pi}{3})$와 $(4, \frac{2\pi}{3})$ 사이의 거리를 구하여라.
먼저, 두 극좌표계에서 정의된 점을 직교좌표계로 변환한다.
1). $(2, \frac{\pi}{3})$
$$\begin{cases} x &= r\cos(\theta) = 2 \cdot \cos(\frac{\pi}{3}) = 2 \cdot \frac{1}{2} = 1 \\ y &= r\sin(\theta) = 2 \cdot \sin(\frac{\pi}{3}) = 2 \cdot \frac{\sqrt{3}}{2} = \sqrt{3} \end{cases}$$
따라서, $(2, \frac{\pi}{3})$의 직교좌표계는 $(1, \sqrt{3})$이다.
2). $(4, \frac{2\pi}{3})$
$$\begin{cases} x &= r\cos(\theta) = 4 \cdot \cos(\frac{2\pi}{3}) = 4 \cdot (-\frac{1}{2}) = -2 \\ y &= r\sin(\theta) = 4 \cdot \sin(\frac{2\pi}{3}) = 4 \cdot \frac{\sqrt{3}}{2} = 2\sqrt{3} \end{cases}$$
따라서, $(4, \frac{2\pi}{3})$의 직교좌표계는 $(-2, 2\sqrt{3})$이다.
1)과 2)의 결과를 이용하여 두 점 사이의 거리를 구하는 공식을 사용하면 된다.
$$D = \sqrt{(1 + 2)^{2} + (\sqrt{3} - 2\sqrt{3})^{2}} = \sqrt{9 + 3} = \sqrt{12} = 2\sqrt{3}$$
연습문제4. 극좌표계에서 정의된 두 점 $(r_{1}, \theta_{1})$와 $(r_{2}, \theta_{2})$ 사이의 거리를 구하여라.
극좌표계에서 직교좌표계로 변환하면 각 점은 $(r_{1}\cos(\theta_{1}), r_{1}\sin(\theta_{1}))$과 $(r_{2}\cos(\theta_{2}), r_{2}\sin(\theta_{2}))$이다. 따라서, 두 점 사이의 거리를 구하는 공식을 이용하면 다음과 같다.
$$\begin{align*} d^{2} &= (r_{1}\cos(\theta_{1}) - r_{2}\cos(\theta_{2}))^{2} + (r_{1}\sin(\theta_{1}) - r_{2}\sin(\theta_{2}))^{2} \\ &= (r^{2}_{1}\cos^{2} (\theta_{1}) - 2r_{1}r_{2}\cos(\theta_{1})\cos(\theta_{2}) + r^{2}_{2}\cos^{2} (\theta_{2}) ) \\ &+ (r^{2}_{1}\sin^{2} (\theta_{1}) - 2r_{1}r_{2}\sin(\theta_{1})\sin(\theta_{2}) + r^{2}_{2}\sin^{2} (\theta_{2})) \\ &= r^{2}_{1} (\sin^{2} (\theta_{1}) + \cos^{2} (\theta_{1})) - 2r_{1}r_{2} (\sin(\theta_{1})\sin(\theta_{2}) + \cos(\theta_{1})\cos(\theta_{2})) + r^{2}_{2} (\sin^{2} (\theta_{2}) + \cos^{2} (\theta_{2})) \\ &= r^{2}_{1} + r^{2}_{2} - 2r_{1}r_{2} \cos(\theta_{1} - \theta_{2}) \end{align*}$$
최종적으로 두 점 사이의 거리는 $d = \sqrt{r^{2}_{1} + r^{2}_{2} - 2r_{1}r_{2}\cos(\theta_{1} - \theta_{2})}$이다.
연습문제5. 극좌표계에서 정의된 곡선들을 직교좌표계로 변환하라.
(a). $r = 2$
(b). $r\cos(\theta) = 1$
(c). $r = 3\sin(\theta)$
(d). $r = 2\sin(\theta) + 2\cos(\theta)$
(e). $r = \csc(\theta)$
(f). $r = \tan(\theta) \sec(\theta)$
(a). $r = 2$
$$r = 2 \Rightarrow x^{2} + y^{2} = 4$$
(b). $r\cos(\theta) = 1$
$$r\cos(\theta) = 1 \Rightarrow x = 1$$
(c). $r = 3\sin(\theta)$
$$r = 3\sin(\theta) \Rightarrow r^{2} = 3r\sin(\theta) \Rightarrow x^{2} + y^{2} = 3y \Rightarrow x^{2} + (y - \frac{3}{2})^{2} = \frac{9}{4}$$
(d). $r = 2\sin(\theta) + 2\cos(\theta)$
$$r = 2\sin(\theta) + 2\cos(\theta) \Rightarrow r^{2} = 2r\sin(\theta) + 2r\cos(\theta) \Rightarrow x^{2} + y^{2} = 2y + 2x \Rightarrow (x - 1)^{2} + (y - 1)^{2} = 2$$
(e). $r = \csc(\theta)$
$$r = \csc(\theta) \Rightarrow r\sin(\theta) = 1 \Rightarrow y = 1$$
(f). $r = \tan(\theta) \sec(\theta)$
$$r = \tan(\theta) \sec(\theta) = \frac{\sin(\theta)}{\cos^{2} (\theta)} \Rightarrow r^{2}\cos^{2} (\theta) = r\sin(\theta) \Rightarrow x^{2} = y$$
연습문제6. 직교좌표계에서 정의된 곡선들을 극좌표계로 변환하라.
(a). $x = 2$
(b). $x^{2} + y^{2} = 9$
(c). $x = -y^{2}$
(d). $x + y = 9$
(e). $x^{2} + y^{2} = 2cx$
(f). $xy = 4$
(a). $x = 2$
$$x = 2 \Rightarrow r\cos(\theta) = 2$$
(b). $x^{2} + y^{2} = 9$
$$x^{2} + y^{2} = 9 \Rightarrow r^{2} = 9 \Rightarrow r = 3$$
(c). $x = -y^{2}$
$$x = -y^{2} \Rightarrow r \cos(\theta) = -r^{2}\sin(\theta) \Rightarrow r\tan(\theta) = -1$$
(d). $x + y = 9$
$$x + y = 9 \Rightarrow r \cos(\theta) + r\sin(\theta) = r(\cos(\theta) + \sin(\theta)) = 9$$
(e). $x^{2} + y^{2} = 2cx$
$$x^{2} + y^{2} = 2cx \Rightarrow r^{2} = 2rc\cos(\theta) \Rightarrow r = 2c\cos(\theta)$$
(f). $xy = 4$
$$xy = 4 \Rightarrow r\cos(\theta) r\sin(\theta) = r^{2}\cos(\theta)\sin(\theta) = 4 \Rightarrow r^{2}\sin(2\theta) = 8$$
참고자료 및 그림출처
Calculus(J. Stewart)
'수학 > 미적분학' 카테고리의 다른 글
| 미적분학 - 극좌표계 미분과 적분 (0) | 2022.04.10 |
|---|---|
| 미적분학 - 극좌표계 곡선 (0) | 2022.04.09 |
| 미적분학 - 매개변수와 미적분학 (0) | 2022.04.06 |
| 미적분학 - 매개변수 함수 (0) | 2022.04.02 |
| 미적분학 - 일 (0) | 2022.03.30 |
