안녕하세요. 지난 포스팅의 미적분학 - 복잡한 삼각함수 적분 2에서는 $\int \tan^{m}(x) \sec^{n}(x) \; dx$ 꼴의 적분을 몇몇 케이스와 예제로 나누어 적분을 해보았습니다. 이전과 마찬가지로 핵심은 삼각함수와 관련된 항등식을 적절하게 활용하는 것이였습니다. 오늘은 치환적분을 통해서 삼각함수를 적분해야하는 경우에 대해서 설명드리도록 하겠습니다.
간단한 예제로 $\int \frac{\sqrt{9 - x^{2}}}{x^{2}} \; dx$를 구해보도록 하겠습니다. 언뜻보면 삼각함수와 관련없어 보이지만 $x = 3\sin(\theta)$라고 두면 $\sqrt{9 - x^{2}} = \sqrt{9 - 9\sin^{2}(\theta)} = 3\sqrt{1 - \sin^{2}(\theta)} = 3 \left|\cos(\theta)\right| = 3\cos(\theta)$가 됩니다. 또한, $dx = 3\cos(\theta) d\theta$입니다. 따라서 아래와 같이 적분 가능합니다.
$$\begin{align*} \int \frac{\sqrt{9 - x^{2}}}{x^{2}} &= \int \frac{3\cos(\theta)}{9\sin^{2}(\theta)}3\cos(\theta) \theta \\ &= \int \frac{\cos^{2}(\theta)}{\sin^{2}(\theta)} \; d\theta \\ &= \int \cot^{2}(\theta) \; d\theta \\ &= \int \left(\csc^{2}(\theta) - 1\right) \; d\theta \\ &= -\cot(\theta) - \theta + C\end{align*}$$
이때, $\cot(\theta) = \frac{\sqrt{9 - x^{2}}}{x}$이고 $\sin(\theta) = \frac{x}{3} \Rightarrow \theta = \arcsin(\frac{x}{3})$ 이기 때문에 최종적으로 아래의 결과를 얻을 수 있습니다.
$$\begin{align*} \int \frac{\sqrt{9 - x^{2}}}{x^{2}} &= -\cot(\theta) - \theta + C \\ &= \frac{\sqrt{9 - x^{2}}}{x} - \arcsin(\frac{x}{3}) + C\end{align*}$$
다음 예제로 $\int \frac{1}{x^{2}\sqrt{x^{2} + 4}} \; dx$를 구해보도록 하겠습니다. 이 역시 언뜻보면 삼각함수와 큰 관련은 없어보입니다. 하지만, $x = 2\tan(\theta) \Rightarrow dx = \sec^{2}(\theta) d\theta$를 얻을 수 있습니다. 이제 식을 변형해보도록 하겠습니다.
$$\frac{1}{x^{2}\sqrt{x^{2} + 4}} = \frac{1}{4\tan^{2}(\theta)\sqrt{4\tan^{2}(\theta) + 4}} = \frac{1}{8\tan^{2}(\theta) \sec(\theta)}$$
따라서 아래와 같이 적분할 수 있습니다.
$$\begin{align*} \int \frac{1}{x^{2}\sqrt{x^{2} + 4}} \; dx &= \int \frac{1}{8\tan^{2}(\theta)\sec(\theta)} \; (2\sec^{2}(\theta) du) \\ &= \frac{1}{4} \int \frac{\sec(\theta)}{\tan^{2}(\theta)} \; d\theta\end{align*}$$
저희는 여기서 추가적으로 식을 변형할 수 있습니다.
$$\frac{\sec(\theta)}{\tan^{2}(\theta)} = \frac{1}{\cos(\theta)} \cdot \frac{\cos^{2}(\theta)}{\sin^{2}(\theta)} = \frac{\cos(\theta)}{\sin^{2}(\theta)}$$
그러므로 다시 적분식을 정리하도록 하겠습니다.
$$\begin{align*} \int \frac{1}{x^{2}\sqrt{x^{2} + 4}} \; dx &= \frac{1}{4} \int \frac{\sec(\theta)}{\tan^{2}(\theta)} \; d\theta \\ &= \frac{1}{4} \int \frac{\cos(\theta)}{\sin^{2}(\theta)} \; d\theta \end{align*}$$
이제, 저희는 $\sin(\theta) = u$라고 하면 $\cos(\theta) d\theta = du \Rightarrow d\theta = \frac{1}{\cos(\theta)} du$를 얻을 수 있습니다. 그러면 다시 적분을 진행해보도록 하겠습니다.
$$\begin{align*} \int \frac{1}{x^{2}\sqrt{x^{2} + 4}} \; dx &= \frac{1}{4} \int \frac{\cos(\theta)}{\sin^{2}(\theta)} \; d\theta \\ &= \frac{1}{4} \int \frac{\cos(\theta)}{\sin^{2}(\theta)} \\ &= \frac{1}{4} \int \frac{\cos(\theta)}{u^{2}} \; \left(\frac{1}{\cos(\theta)} du\right) = \frac{1}{4} \int \frac{1}{u^{2}} \; du \\ &= \frac{1}{4} \left(-\frac{1}{u}\right) + C \\ &= -\frac{1}{4\sin(\theta)} + C\end{align*}$$
마지막으로 저희가 $x = 2\tan(\theta)$로 치환했기 때문에 $\sin(\theta) = \frac{x}{\sqrt{x^{2} + 4}}$를 얻을 수 있습니다. 따라서, 마지막 식은 아래와 같습니다.
$$\begin{align*} \int \frac{1}{x^{2}\sqrt{x^{2} + 4}} \; dx &= -\frac{1}{4\sin(\theta)} + C \\ &= -\frac{\sqrt{x^{2} + 4}}{4x} + C \end{align*}$$
연습문제1. 주어진 적분을 계산하라.
(a). $\int_{0}^{2\sqrt{3}} \frac{x}{\sqrt{16 - x^{2}}} \; dx$
(b). $\int_{\sqrt{2}}^{2} \frac{1}{x^{3}\sqrt{x^{2} - 1}} \; dx$
(c). $\int_{1}^{2} \frac{\sqrt{x^{2} - 1}}{x} \; dx$
(d). $\int \frac{1}{x^{2}\sqrt{25 - x^{2}}} \; dx$
(e). $\int \frac{x^{3}}{\sqrt{x^{2} + 100}} \; dx$
(f). $\int \frac{1}{\sqrt{x^{2} + 16}} \; dx$
(g). $\int \frac{x^{5}}{\sqrt{x^{2} + 2}} \; dx$
(a). $\int_{0}^{2\sqrt{3}} \frac{x}{\sqrt{16 - x^{2}}} \; dx$
$x = 4\sin(\theta)$라고 하면 $dx = 4\cos(\theta) d\theta$이고 적분구간은 $[0, 2\sqrt{3}] \xrightarrow{\theta = \arcsin(\frac{x}{4})} [\arcsin(0), \arcsin(\frac{\sqrt{3}}{2})] = [0, \frac{\pi}{3}]$이다.
$$\begin{align*} \int_{0}^{2\sqrt{3}} \frac{x}{\sqrt{16 - x^{2}}} \; dx &= \int_{0}^{\frac{\pi}{3}} \frac{4sin(\theta)}{\sqrt{16 - 16\sin^{2}(\theta)}} \cdot 4\cos(\theta) d\theta \\ &= \int_{0}^{\frac{\pi}{3}} \frac{16\sin(\theta)\cos(\theta)}{4\cos(\theta)} \; d\theta \\ &= \int_{0}^{\frac{\pi}{3}} 4\sin(\theta) \; d\theta \\ &= [-4\cos(\theta)]_{0}^{\frac{\pi}{3}} \\ &= -4(\cos(\frac{\pi}{3}) - \cos(0)) \\ &= -4(\frac{1}{2} - 1) = 2 \end{align*}$$
(b). $\int_{\sqrt{2}}^{2} \frac{1}{x^{3}\sqrt{x^{2} - 1}} \; dx$
$x = \sec(\theta)$라고 하면 $dx = \sec(\theta)\tan(\theta) d\theta$이고 적분구간은 $[\sqrt{2}, 2] \xrightarrow{\theta = \arcsec(x)} [\arcsin(\frac{1}{\sqrt{2}}), \arcsin(\frac{1}{2})] = [\frac{\pi}{4}, \frac{\pi}{3}]$이다.
$$\begin{align*} \int_{\sqrt{2}}^{2} \frac{1}{x^{3}\sqrt{x^{2} - 1}} \; dx &= \int_{\frac{\pi}{4}}^{\frac{\pi}{3}} \frac{1}{\sec^{3}(\theta) \sqrt{\sec^{2}(\theta) - 1}} \cdot \sec(\theta)\tan(\theta) d\theta \\ &= \int_{\frac{\pi}{4}}^{\frac{\pi}{3}} \frac{\sec(\theta)\tan(\theta)}{\sec^{3}(\theta)\tan(\theta)} \; d\theta \\ &= \int_{\frac{\pi}{4}}^{\frac{\pi}{3}} \cos^{2}(\theta) \; d\theta \\ &= \int_{\frac{\pi}{4}}^{\frac{\pi}{3}} \left( \frac{1 + 2\cos(2\theta)}{2} \right) \; d\theta \\ &= \frac{1}{2}\int_{\frac{\pi}{4}}^{\frac{\pi}{3}} (1 + 2\cos(2\theta)) \; d\theta \\ &= \frac{1}{2} \left[ \theta + \sin(2\theta) \right]_{\frac{\pi}{4}}^{\frac{\pi}{3}} \\ &= \frac{1}{2} \left[ \left( \frac{\pi}{3} + \sin(\frac{2\pi}{3}) \right) - \left( \frac{\pi}{4} + \sin(\frac{\pi}{2}) \right) \right] = \frac{1}{2} \left( \frac{\pi}{12} + \frac{\sqrt{3}}{2} - 1 \right)\end{align*}$$
(c). $\int_{1}^{2} \frac{\sqrt{x^{2} - 1}}{x} \; dx$
$x = \sec(\theta)$라고 하면 $dx = \sec(\theta)\tan(\theta) d\theta$이고 적분구간은 $[1, 2] \xrightarrow{\theta = \arcsec(x)} [\arcsin(1), \arcsin(\frac{1}{2})] = [0, \frac{\pi}{3}]$이다.
$$\begin{align*} \int_{1}^{2} \frac{x^{2} - 1}{x} \; dx &= \int_{0}^{\frac{\pi}{3}} \frac{\sqrt{\sec^{2}(\theta) - 1}}{\sec(\theta)} \cdot (\sec(\theta)\tan(\theta)) \; d\theta \\ &= \int_{0}^{\frac{\pi}{3}} \frac{\tan(\theta)}{\sec(\theta)} \cdot (\sec(\theta)\tan(\theta)) \; d\theta \\ &= \int_{0}^{\frac{\pi}{3}} \tan^{2}(\theta) \; d\theta \\ &= \int_{0}^{\frac{\pi}{3}} (\sec^{2}(\theta) - 1) \; d\theta \\ &= \left[ \tan(\theta) - \theta \right]_{0}^{\frac{\pi}{3}} \\ &= \left( \tan(\frac{\pi}{3}) - \frac{\pi}{3} \right) - \left( \tan(0) - 0 \right) = \sqrt{3} - \frac{\pi}{3} \end{align*}$$
(d). $\int \frac{1}{x^{2}\sqrt{25 - x^{2}}} \; dx$
$x = 5\sin(\theta)$라고 하면 $dx = 5\cos(\theta) d\theta$이다.
$$\begin{align*} \int \frac{1}{x^{2}\sqrt{25 - x^{2}}} \; dx &= \int \frac{1}{25\sin^{2}(\theta)\sqrt{25 - 25\sin^{2}(\theta)}} \cdot (5\cos(\theta)) \; d\theta \\ &= \int \frac{1}{25\sin^{2}(\theta)} \; d\theta \\ &= \frac{1}{25} \int \csc^{2}(\theta) \; d\theta \\ &= -\frac{1}{25} \cot(\theta) + C \\ &= -\frac{1}{25} \frac{\sqrt{25 - x^{2}}}{x} + C \end{align*}$$
(e). $\int \frac{x^{3}}{\sqrt{x^{2} + 100}} \; dx$
$x = 10\tan(\theta)$라고 하면 $dx = 10\sec^{2}(\theta) d\theta$이다.
$$\begin{align*} \int \frac{x^{3}}{\sqrt{x^{2} + 100}} \; dx &= \int \frac{1000\tan^{3}(\theta)}{\sqrt{100\tan^{2}(\theta) + 1}} \cdot (10\sec^{2}(\theta)) \; d\theta \\ &= 1000\int \tan^{3}(\theta)\sec(\theta) \; d\theta \\ &= 1000 \int \tan^{2}(\theta) \tan(\theta)\sec(\theta) \; d\theta \\ &= 1000 \int (\sec^{2}(\theta) - 1) \tan(\theta)\sec(\theta) \; d\theta \end{align*}$$
여기서 $\sec(\theta) = u$라고 하면 $\sec(\theta)\tan(\theta) d\theta = du \rightarrow d\theta = \frac{1}{\sec(\theta)\tan(\theta)} du$이다.
$$\begin{align*} \int \frac{x^{3}}{\sqrt{x^{2} + 100}} \; dx &= 1000 \int (\sec^{2}(\theta) - 1) \tan(\theta)\sec(\theta) \; d\theta \\ &= 1000 \int (u^{2} - 1) \tan(\theta)\sec(\theta) \cdot \frac{1}{\sec(\theta)\tan(\theta)} \; d\theta \\ &= 1000 \int (u^{2} - 1) \; du \\ &= 1000 \left( \frac{1}{3}u^{3} - u \right) + C \\ &= 1000 \left( \frac{1}{3}\sec^{3}(\theta) - \sec(\theta) \right) + C \\ &= 1000 \left( \frac{(100 + x^{2})\sqrt{100 + x^{2}}}{3000} - \frac{\sqrt{100 + x^{2}}}{10} \right) + C \end{align*}$$
(f). $\int \frac{1}{\sqrt{x^{2} + 16}} \; dx$
$x = 4\tan(\theta)$라고 하면 $dx = 4\sec^{2}(\theta) d\theta$이다.
$$\begin{align*} \int \frac{1}{\sqrt{x^{2} + 16}} \; dx &= \int \frac{1}{\sqrt{16\tan^{2}(\theta) + 16}} \cdot (4\sec^{2}(\theta)) \; d\theta \\ &= \int \sec(\theta) \; d\theta \\ &= \ln|\sec(\theta) + \tan(\theta)| + C \\ &= \ln \left| \frac{\sqrt{16 + x^{2}}}{4} + \frac{x}{4} \right| + C \end{align*}$$
(g). $\int \frac{x^{5}}{\sqrt{x^{2} + 2}} \; dx$
$x = \sqrt{2}\tan(\theta)$라고 하면 $dx = \sqrt{2}\sec^{2}(\theta) d\theta$이다.
$$\begin{align*} \int \frac{x^{5}}{\sqrt{x^{2} + 2}} \; dx &= \int \frac{4\sqrt{2}\tan^{5}(\theta)}{\sqrt{2} \sec(\theta)} \cdot (\sqrt{2} \sec^{2}(\theta)) \; d\theta \\ &= 4\sqrt{2} \int \tan^{5}(\theta)\sec(\theta) \; d\theta \\ &= 4\sqrt{2} \int \tan^{4}(\theta) \tan(\theta)\sec(\theta) \; d\theta \\ &= 4\sqrt{2} \int (\sec^{2}(\theta) - 1)^{2} \tan(\theta)\sec(\theta) \; d\theta \end{align*}$$
여기서 $\sec(\theta) = u$라고 하면 $\sec(\theta)\tan(\theta) d\theta = du \rightarrow d\theta = \frac{1}{\sec(\theta)\tan(\theta)} du$이다.
$$\begin{align*} \int \frac{x^{5}}{\sqrt{x^{2} + 2}} \; dx &= 4\sqrt{2} \int (\sec^{2}(\theta) - 1)^{2} \tan(\theta)\sec(\theta) \; d\theta \\ &= 4\sqrt{2} \int (u^{2} - 1)^{2} \; du \\ &= 4\sqrt{2} \int (u^{4} - 2u^{2} + 1) \; du \\ &= 4\sqrt{2} \left( \frac{1}{5}u^{5} - \frac{2}{3}u^{3} + u \right) + C \\ &= 4\sqrt{2} \left( \frac{1}{5}\sec^{5}(\theta) - \frac{2}{3}\sec^{3}(\theta) + \sec(\theta) \right) + C \\ &= 4\sqrt{2} \left( \frac{(x^{2} + 2)^{2}\sqrt{x^{2} + 2}}{4\sqrt{2}} - \frac{2}{3} \frac{(x^{2} + 2)\sqrt{x^{2} + 2}}{2} + \frac{\sqrt{x^{2} + 2}}{\sqrt{2}} \right) + C \end{align*}$$
연습문제2. 주어진 적분을 계산하라.
(a). $\int \sqrt{1 - 4x^{2}} \; dx$
(b). $\int_{0}^{1} x\sqrt{x^{2} + 4} \; dx$
(c). $\int \frac{\sqrt{x^{2} - 9}}{x^{3}} \; dx$
(d). $\int \frac{1}{x\sqrt{5 - x^{2}}} \; dx$
(e). $\int_{0}^{a} x^{2}\sqrt{a^{2} - x^{2}} \; dx$
(a). $\int \sqrt{1 - 4x^{2}} \; dx$
$x = \frac{1}{2}\sin(\theta)$라고 하면 $dx = \frac{1}{2}\cos(\theta) d\theta$이다.
$$\begin{align*} \int \sqrt{1 - 4x^{2}} \; dx &= \int \sqrt{1 - \sin^{2}(\theta)} \cdot (\frac{1}{2}\cos(\theta)) \; d\theta \\ &= \frac{1}{2} \int \cos^{2}(\theta) \; d\theta \\ &= \frac{1}{4} \int \left( 1 + \cos(2\theta) \right) \; d\theta \\ &= \frac{1}{4} \left( \theta + \frac{1}{2} \sin(2\theta) \right) + C \\ &= \frac{1}{4} (\arcsin(2x) + \frac{1}{2} (\sqrt{1 - 4x^{2}} - 4x^{2}) ) + C \end{align*}$$
(b). $\int_{0}^{1} x\sqrt{x^{2} + 4} \; dx$
$x = 2\tan(\theta)$라고 하면 $dx = 2\sec^{2}(\theta) d\theta$이고적분구간은 $[0, 1] \xrightarrow{\theta = \arctan(\frac{x}{2})} [\arctan(0), \arctan(\frac{1}{2})] = [0, \alpha]$이다. 여기서, $\alpha = \arctan(\frac{1}{2})$이다.
$$\begin{align*} \int_{0}^{1} x\sqrt{x^{2} + 4} \; dx &= \int_{0}^{\alpha} 2\tan(\theta) \sqrt{4\tan^{2}(\theta) + 4} \cdot (2\sec^{2}(\theta)) \; d\theta \\ &= 8\int_{0}^{\alpha} \tan(\theta) \sec^{3}(\theta) \; d\theta \end{align*}$$
여기서, $\sec(\theta) = u$라고 하면 $\sec(\theta) \tan(\theta) d\theta = du \rightarrow d\theta = \frac{1}{\sec(\theta)\tan(\theta)} du$이고 적분구간은 $[0, \alpha] \xrightarrow{u = \sec(\theta)} [\sec(0), \sec(\alpha)] = [1, \sec(\alpha)]$이다.
$$\begin{align*} \int_{0}^{1} x\sqrt{x^{2} + 4} \; dx &= 8\int_{0}^{\alpha} \tan(\theta) \sec^{3}(\theta) \; d\theta \\ &= 8\int_{1}^{\sec(\alpha)} u^{2} \sec(\theta)\tan(\theta) \cdot \frac{1}{\sec(\theta)\tan(\theta)} \; du \\ &= 8\int_{1}^{\sec(\alpha)} u^{2} \; du \\ &= \left[ \frac{1}{3}u^{3} \right]_{1}^{\sec(\alpha)} = \frac{8}{3} \left(\sec^{3}(\alpha) - 1\right) \end{align*}$$
(c). $\int \frac{\sqrt{x^{2} - 9}}{x^{3}} \; dx$
$x = 3\sec(\theta)$라고 하면 $dx = 3\sec(\theta)\tan(\theta) d\theta$이다.
$$\begin{align*} \int \frac{\sqrt{x^{2} - 9}}{x^{3}} \; dx &= \int \frac{\sqrt{9\sec^{2}(\theta) - 9}}{27\sec^{3}(\theta)} \cdot (3\sec(\theta)\tan(\theta)) \; d\theta \\ &= \int \frac{3\tan(\theta)}{27\sec^{3}(\theta)} \cdot (3\sec(\theta)\tan(\theta)) \; d\theta \\ &= \frac{1}{3} \int \frac{\tan^{2}(\theta)}{\sec^{2}(\theta)} \; d\theta \\ &= \frac{1}{3} \int \sin^{2}(\theta) \; d\theta \\ &= \frac{1}{6} \int (1 - \cos(2\theta)) \; d\theta \\ &= \frac{1}{6} \left( \theta - \frac{1}{2}\sin(2\theta) \right) + C \\ &= \frac{1}{6} \left( \arcsec(\frac{x}{3}) - \frac{6\sqrt{x^{2} - 9}}{x} \right) + C \end{align*}$$
(d). $\int \frac{1}{x\sqrt{5 - x^{2}}} \; dx$
$x = \sqrt{5}\sin(\theta)$라고 하면 $dx = \sqrt{5}\cos(\theta) d\theta$이다.
$$\begin{align*} \int \frac{1}{x\sqrt{5 - x^{2}}} \; dx &= \int \frac{1}{\sqrt{5}\sin(\theta) \sqrt{5 - 5\sin^{2}(\theta)}} \cdot (\sqrt{5}\cos(\theta)) \; d\theta \\ &= \frac{1}{\sqrt{5}} \int \frac{1}{\sin(\theta)} \; d\theta \\ &= \frac{1}{\sqrt{5}} \ln \left| \frac{1}{\csc(\theta) + \cot(\theta)} \right| + C \\ &= \frac{1}{\sqrt{5}} \ln \left| \frac{x}{\sqrt{5 - x^{2}} + \sqrt{5}} \right| + C \end{align*}$$
(e). $\int_{0}^{a} x^{2}\sqrt{a^{2} - x^{2}} \; dx$
$x = a\sin(\theta)$라고 하면 $dx = a\cos(\theta) d\theta$이고 적분구간은 $[0, a] \xrightarrow{\theta = \arcsin(\frac{x}{a})} [\arcsin(0), \arcsin(1)] = [0, \frac{\pi}{2}] $이다.
$$\begin{align*} \int_{0}^{a} x^{2}\sqrt{a^{2} - x^{2}} \; dx &= \int_{0}^{\frac{\pi}{2}} a^{2}\sin^{2}(\theta) \sqrt{a^{2} - a^{2}\sin^{2}(\theta)} \cdot (a\cos(\theta))\; d\theta \\ &= a^{4} \int_{0}^{\frac{\pi}{2}} (\sin(\theta) \cos(\theta))^{2} \; d\theta \\ &= \frac{a^{4}}{4} \int_{0}^{\frac{\pi}{2}} \sin^{2}(2\theta) \; d\theta \\ &= \frac{a^{4}}{8} \int_{0}^{\frac{\pi}{2}} (1 - \cos(4\theta)) \; d\theta \\ &= \frac{a^{4}}{8} \left[ \theta - \frac{1}{4}\sin(4\theta) \right]_{0}^{\frac{\pi}{2}} = \frac{a^{4}\pi}{16} \end{align*}$$
연습문제3. 주어진 적분을 계산하라.
(a). $\int \frac{x}{\sqrt{x^{2} - 7}} \; dx$
(b). $\int \frac{\sqrt{1 + x^{2}}}{x} \; dx$
(c). $\int \frac{x}{\sqrt{25 - x^{2}}} \; dx$
(d). $\int_{0}^{1} \sqrt{x^{2} + 1} \; dx$
(e). $\int \sqrt{5 + 4x - x^{2}} \; dx$
(a). $\int \frac{x}{x^{2} - 7} \; dx$
$x = \sqrt{7}\sec(\theta)$라고 하면 $dx = \sqrt{7}\sec(\theta)\tan(\theta) d\theta$이다.
$$\begin{align*} \int \frac{x}{\sqrt{x^{2} - 7}} \; dx &= \int \frac{\sqrt{7}\sec(\theta)}{\sqrt{7\sec^{2}(\theta) - 7}} \cdot (\sqrt{7}\sec(\theta)\tan(\theta)) \; d\theta \\ &= \int \sqrt{7}\sec^{2}(\theta) \; d\theta \\ &= \sqrt{7}\tan(\theta) + C \\ &= \sqrt{7} \cdot \frac{\sqrt{x^{2} - 7}}{\sqrt{7}} + C \\ &= \sqrt{x^{2} - 7} + C \end{align*}$$
(b). $\int \frac{\sqrt{1 + x^{2}}}{x} \; dx$
$x = \tan(\theta)$라고 하면 $dx = \sec^{2}(\theta) d\theta$이다.
$$\begin{align*} \int \frac{\sqrt{1 + x^{2}}}{x} \; dx &= \int \frac{\sqrt{1 + \tan^{2}(\theta)}}{\tan(\theta)} \cdot (\sec^{2}(\theta)) \; d\theta \\ &= \int \frac{\sec^{3}(\theta)}{\tan(\theta)} \; d\theta \\ &= \int \frac{(1 + \tan^{2}(\theta))\sec(\theta)}{\tan(\theta)} \; d\theta \\ &= \int \left( \frac{\sec(\theta)}{\tan(\theta)} + \sec(\theta)\tan(\theta) \right) \; d\theta \\ &= \int \left( \csc(\theta) + \tan(\theta)\sec(\theta) \right) \; d\theta \\ &= \ln \left|\frac{1}{\csc(\theta) + \cot(\theta)}\right| + \sec(\theta) + C \\ &= \ln \left| \frac{1}{\sqrt{x^{2} + 1} + \frac{1}{x}} \right| + \frac{\sqrt{x^{2} + 1}}{x} + C \end{align*}$$
(c). $\int \frac{x}{\sqrt{25 - x^{2}}} \; dx$
$x = 5\sin(\theta)$라고 하면 $dx = 5\cos(\theta) d\theta$이다.
$$\begin{align*} \int \frac{x}{\sqrt{25 - x^{2}}} \; dx &= \int \frac{5\sin(\theta)}{\sqrt{25 - 25\sin^{2}(\theta)}} \cdot (5\cos(\theta)) \; d\theta \\ &= \int \frac{5\sin(\theta)}{5\cos(\theta)} \cdot (5\cos(\theta)) \; d\theta \\ &= 5\int \sin(\theta) \; d\theta \\ &= -5\cos(\theta) + C \\ &= -5 \cdot \frac{\sqrt{25 - x^{2}}}{5} = C \\ &= -\sqrt{25 - x^{2}} + C \end{align*}$$
(d). $\int_{0}^{1} \sqrt{x^{2} + 1} \; dx$
$x = \tan(\theta)$라고 하면 $dx = \sec^{2}(\theta) d\theta$이고 적분구간은 $[0, 1] \xrightarrow{\theta = \arctan(x)} [\arctan(0), \arctan(1)] = [0, \frac{\pi}{4}] $이다.
$$\begin{align*} \int_{0}^{1} \sqrt{x^{2} + 1} \; dx &= \int_{0}^{\frac{\pi}{4}} \sqrt{\tan^{2}(\theta) + 1} \cdot (\sec^{2}(\theta)) \; d\theta \\ &= \int_{0}^{\frac{\pi}{4}} \sec^{3}(\theta) \; d\theta \\ &= \int_{0}^{\frac{\pi}{4}} (1 + \tan^{2}(\theta)) \sec(\theta) \; d\theta \\ &= \int_{0}^{\frac{\pi}{4}} \left( \sec(\theta) + \tan^{2}(\theta)\sec(\theta) \right) \; d\theta \end{align*}$$
1). $\int_{0}^{\frac{\pi}{4}} \sec(\theta) \; d\theta = \left[ \ln|\sec(\theta) + \tan(\theta)| \right]_{0}^{\frac{\pi}{4}} = \ln (\sqrt{2} + 1)$
2). $f = \tan(\theta)$ 그리고 $g^{'} = \tan(\theta)\sec(\theta)$라고 하면 $f^{'} = \sec^{2}(\theta)$ 이고 $g = \sec(\theta)$이다.
$$\begin{align*} \int_{0}^{\frac{\pi}{4}} \tan^{2}(\theta)\sec(\theta) \; d\theta &= \left[ \tan(\theta)\sec(\theta) \right]_{0}^{\frac{\pi}{4}} - \int_{0}^{\frac{\pi}{4}} \sec^{3}(\theta) \; d\theta \\ &= \sqrt{2} - \int_{0}^{\frac{\pi}{4}} \sec^{3}(\theta) \; d\theta \end{align*}$$
이제 식을 정리하면 다음과 같다.
$$\begin{align*} \int_{0}^{1} \sqrt{x^{2} + 1} \; dx &= \int_{0}^{\frac{\pi}{4}} \left( \sec(\theta) + \tan^{2}(\theta)\sec(\theta) \right) \; d\theta \\ &= \ln (\sqrt{2} + 1) + \sqrt{2} - \int_{0}^{\frac{\pi}{4}} \sec^{3}(\theta) \; d\theta \\ \Rightarrow& \int_{0}^{1} \sqrt{x^{2} + 1} \; dx = \int_{0}^{\frac{\pi}{4}} \sec^{3}(\theta) \; d\theta = \frac{1}{2} \left( \ln (\sqrt{2} + 1) + \sqrt{2} \right) \end{align*}$$
(e). $\int \sqrt{5 + 4x - x^{2}} \; dx$
먼저, 식을 완전제곱식으로 바꾸면 $5 + 4x - x^{2} = 9 - (x^{2} - 4x + 4) = 9 - (x - 2)^{2}$이다. 이제, $x - 2 = u$라고 두면 다음과 같이 적분할 수 있다.
$$\begin{align*} \int \sqrt{5 + 4x - x^{2}} \; dx &= \int \sqrt{9 - (x - 2)^{2}} \; dx \\ &= \int \sqrt{9 - u^{2}} \; du \end{align*}$$
이제, $u = 3\sin(\theta)$라고 두면 $du = 3\cos(\theta)$이다.
$$\begin{align*} \int \sqrt{5 + 4x - x^{2}} \; dx &= \int \sqrt{9 - u^{2}} \; du \\ &= \int \sqrt{9 - 9\sin^{2}(\theta)} \cdot (3\cos(\theta)) \; d\theta \\ &= \int 9\cos^{2}(\theta) \; d\theta \\ &= \frac{9}{2} \int \left( 1 + \cos(2\theta) \right) \; d\theta \\ &= \frac{9}{2} \left( \theta + \frac{1}{2}\sin(2\theta) \right) + C \\ &= \frac{9}{2} \left( \arcsin \left( \frac{u}{3} \right) + \frac{1}{2} \frac{u\sqrt{9 - u^{2}}}{9} \right) + C \\ &= \frac{9}{2} \left( \arcsin \left( \frac{1}{3}(x - 2) \right) + \frac{(x - 2)\sqrt{5 + 4x - x^{2}}}{18} \right) + C \end{align*}$$
연습문제4. 주어진 적분을 계산하라.
(a). $\int \frac{x}{\sqrt{x^{2} + x + 1}} \; dx$
(b). $\int \frac{x^{2}}{(3 + 4x - 4x^{2})^{\frac{3}{2}}} \; dx$
(c). $\int \sqrt{x^{2} + 2x} \; dx$
(d). $\int \frac{x^{2} + 1}{(x^{2} - 2x + 2)^{2}} \; dx$
(e). $\int x\sqrt{1 - x^{4}} \; dx$
(a). $\int \frac{x}{\sqrt{x^{2} + x + 1}} \; dx$
먼저, 식을 완전제곱식으로 바꾸면 $\frac{x}{\sqrt{x^{2} + x + 1}} = \frac{x}{\sqrt{(x + \frac{1}{2})^{2} + \frac{3}{4}}}$이다. 이제, $x + \frac{1}{2} = u$라고 두면 다음과 같이 적분할 수 있다.
$$\begin{align*} \int \frac{x}{\sqrt{x^{2} + x + 1}} \; dx &= \int \frac{x}{\sqrt{(x + \frac{1}{2})^{2} + \frac{3}{4}}} \; dx \\ &= \int \frac{u - \frac{1}{2}}{\sqrt{u^{2} +\frac{3}{4}}} \; du \\ &= \int \left( \frac{u}{\sqrt{u^{2} + \frac{3}{4}}} - \frac{1}{2} \cdot \frac{1}{\sqrt{u^{2} + \frac{3}{4}}} \right) \; du\end{align*}$$
1). $\int \frac{u}{\sqrt{u^{2} + \frac{3}{4}}} \; du$
먼저, $u^{2} +\frac{3}{4} = v$라고 두면 $2u du = dv \rightarrow du = \frac{1}{2u} dv$이다.
$$\begin{align*} \int \frac{u}{\sqrt{u^{2} + \frac{3}{4}}} \; du &= \int \frac{u}{\sqrt{v}} \cdot \frac{1}{2u} \; dv \\ &= \frac{1}{2} \int v^{-\frac{1}{2}} \; dv \\ &= \sqrt{v} + C_{1} \\ &= \sqrt{u^{2} + \frac{3}{4}} + C_{1} \\ &= \sqrt{x^{2} + x + 1} + C_{1} \end{align*}$$
2). $\int \frac{1}{\sqrt{u^{2} + \frac{3}{4}}} \; du$
먼저, $u = \frac{\sqrt{3}}{2} \tan(\theta)$라고 두면 $du = \frac{\sqrt{3}}{2} \sec^{2}(\theta) d\theta$이다.
$$\begin{align*} \int \frac{1}{\sqrt{u^{2} + \frac{3}{4}}} \; du &= \int \frac{1}{\sqrt{\frac{3}{4}\tan^{2}(\theta) + \frac{3}{4}}} \cdot \frac{\sqrt{3}}{2}\sec^{2}(\theta) \; d\theta \\ &= \int \sec(\theta) \; d\theta \\ &= \ln |\sec(\theta) + \tan(\theta)| + C_{2} \\ &= \ln \left| \frac{\sqrt{4u^{2} + 3} + 2u}{\sqrt{3}} \right| + C_{2} \\ &= \ln \left| \frac{2\left( \sqrt{x^{2} + x + 1} + (x + \frac{1}{2}) \right)}{\sqrt{3}} \right| + C_{2} \end{align*}$$
1)과 2)의 적분결과에 의해 기존 적분식은 다음과 같이 풀 수 있다.
$$\begin{align*} \int \frac{x}{\sqrt{x^{2} + x + 1}} \; dx &= \int \frac{x}{\sqrt{(x + \frac{1}{2})^{2} + \frac{3}{4}}} \; dx \\ &= \int \frac{u - \frac{1}{2}}{\sqrt{u^{2} +\frac{3}{4}}} \; du \\ &= \int \left( \frac{u}{\sqrt{u^{2} + \frac{3}{4}}} - \frac{1}{2} \cdot \frac{1}{\sqrt{u^{2} + \frac{3}{4}}} \right) \; du \\ &= \sqrt{x^{2} + x + 1} + \ln \left| \frac{2}{\sqrt{3}}\left( \sqrt{x^{2} + x + 1} + (x + \frac{1}{2}) \right) \right| + C \end{align*}$$
(b). $\int \frac{x^{2}}{(3 + 4x - 4x^{2})^{\frac{3}{2}}} \; dx$
먼저, 식을 완전제곱식으로 바꾸면 $\frac{x^{2}}{(3 + 4x - 4x^{2})^{\frac{3}{2}}} = \frac{x^{2}}{(4 - (2x - 1)^{2})^{\frac{3}{2}}}$이다. 이제, $2x - 1 = u$라고 두면 다음과 같이 적분할 수 있다.
$$\begin{align*} \int \frac{x^{2}}{(3 + 4x - 4x^{2})^{\frac{3}{2}}} \; dx &= \int \frac{x^{2}}{(4 - (2x - 1)^{2})^{\frac{3}{2}}} \; dx \\ &= \int \frac{\frac{1}{4}(u + 1)^{2}}{(4 - u^{2})^{\frac{3}{2}}} \; du \\ &= \frac{1}{4} \int \frac{u^{2} + 2u + 1}{(4 - u^{2})^{\frac{3}{2}}} \; du \\ &= \frac{1}{4} \int \left( \frac{u^{2}}{(4 - u^{2})^{\frac{3}{2}}} + 2\frac{u}{(4 - u^{2})^{\frac{3}{2}}} + \frac{1}{(4 - u^{2})^{\frac{3}{2}}} \right) \; du \end{align*}$$
1). $\int \frac{u^{2}}{(4 - u^{2})^{\frac{3}{2}}} \; du$
$u = 2\sin(\theta)$라고 두면 $du = 2\cos(\theta) d\theta$이다.
$$\begin{align*} \int \frac{u^{2}}{(4 - u^{2})^{\frac{3}{2}}} \; du \\ &= \int \frac{4\sin^{2}(\theta)}{(4 - 4\sin^{2}(\theta))^{\frac{3}{2}}} \cdot (2\cos(\theta)) \; d\theta \\ &= \int \frac{\sin^{2}(\theta)}{\cos^{2}(\theta)} \; d\theta \\ &= \int \tan^{2}(\theta) \; d\theta \\ &= \int (\sec^{2}(\theta) - 1) \; d\theta \\ &= \tan(\theta) - \theta + C_{1} \\ &= \frac{u}{\sqrt{4 - u^{2}}} - \arcsin(\frac{u}{2}) + C_{1} \\ &= \frac{2x - 1}{\sqrt{3 + 4x - 4x^{2}}} - \arcsin(\frac{1}{2}(2x - 1)) + C_{1} \end{align*}$$
2). $\int \frac{u}{(4 - u^{2})^{\frac{3}{2}}} \; du$
$4 - u^{2} = v$라고 두면 $-2u du = dv \rightarrow du = -\frac{1}{2u} dv$이다.
$$\begin{align*} \int \frac{u}{(4 - u^{2})^{\frac{3}{2}}} \; du &= \int \frac{u}{v^{\frac{3}{2}}} \cdot \left( -\frac{1}{2u} \right) \; dv \\ &= -\frac{1}{2} \int v^{-\frac{3}{2}} \; dv \\ &= -\frac{1}{2} \cdot \frac{1}{1 - \frac{3}{2}} v^{-\frac{1}{2}} + C_{2} \\ &= v^{-\frac{1}{2}} + C_{2} \\ &= \frac{1}{\sqrt{4 - u^{2}}} + C_{2} \\ &= \frac{1}{\sqrt{3 + 4x - x^{2}}} + C_{2} \end{align*}$$
3). $\int \frac{1}{(4 - u^{2})^{\frac{3}{2}}} \; du$
$u = 2\sin(\theta)$라고 두면 $du = 2\cos(\theta) d\theta$이다.
$$\begin{align*} \int \frac{1}{(4 - u^{2})^{\frac{3}{2}}} \; du \\ &= \int \frac{1}{(4 - 4\sin^{2}(\theta))^{\frac{3}{2}}} \cdot (2\cos(\theta)) \; d\theta \\ &= \frac{1}{4} \int \frac{1}{\cos^{2}(\theta)} \; d\theta \\ &= \frac{1}{4} \int \sec^{2}(\theta) \; d\theta \\ &= \frac{1}{4} \tan(\theta) + C_{3} \\ &= \frac{1}{4} \frac{u}{\sqrt{4 - u^{2}}} + C_{3} \\ &= \frac{2x - 1}{4\sqrt{3 + 4x - 4x^{2}}} + C_{3} \end{align*}$$
1), 2), 3)의 적분결과에 의해 기존 적분식은 다음과 같이 풀 수 있다.
$$\begin{align*} \int \frac{x^{2}}{(3 + 4x - 4x^{2})^{\frac{3}{2}}} \; dx &= \frac{1}{4} \int \left( \frac{u^{2}}{(4 - u^{2})^{\frac{3}{2}}} + 2\frac{u}{(4 - u^{2})^{\frac{3}{2}}} + \frac{1}{(4 - u^{2})^{\frac{3}{2}}} \right) \; du \\ &= \frac{1}{4} \left( \frac{2x - 1}{\sqrt{3 + 4x - 4x^{2}}} - \arcsin(\frac{1}{2}(2x - 1)) + \frac{2}{\sqrt{3 + 4x - x^{2}}} + \frac{2x - 1}{4\sqrt{3 + 4x - 4x^{2}}} \right) + C \end{align*}$$
(c). $\int \sqrt{x^{2} + 2x} \; dx$
먼저, 식을 완전제곱식으로 바꾸면 $\sqrt{x^{2} + 2x} = \sqrt{(x^{2} + 2x + 1) - 1} = \sqrt{(x + 1)^{2} - 1}$이다. 이제, $x + 1 = \sec(\theta)$라고 두면 다음과 같이 적분할 수 있다.
$$\begin{align*} \int \sqrt{x^{2} + 2x} \; dx &= \int \sqrt{(x + 1)^{2} - 1} \; dx \\ &= \int \sqrt{\sec^{2}(\theta) - 1} \cdot (\sec(\theta)\tan(\theta)) \; d\theta \\ &= \int \sec(\theta)\tan^{2}(\theta) \; d\theta \end{align*}$$
이제, $f = \tan(\theta), g^{'} = \sec(\theta)\tan(\theta)$라고 하면 $f^{'} = \sec^{2}(\theta), g = \sec(\theta)$이므로 부분적분을 적용하여 다음과 같이 적분할 수 있다.
$$\begin{align*} \int \sqrt{x^{2} + 2x} \; dx &= \int \sec(\theta)\tan^{2}(\theta) \; d\theta \\ &= \tan(\theta)\sec(\theta) - \int \sec^{3}(\theta) \; d\theta \\ &= \tan(\theta)\sec(\theta) - \int \sec(\theta) (1 + \tan^{2}(\theta)) \; d\theta \\ &= \tan(\theta)\sec(\theta) - \ln |\sec(\theta) + \tan(\theta)| - \int \sec(\theta)\tan^{2}(\theta) \; d\theta \\ \Rightarrow& \int \sqrt{x^{2} + 2x} \; dx = \int \sec(\theta)\tan^{2}(\theta) \; d\theta \\ &= \frac{1}{2} \left( \tan(\theta)\sec(\theta) - \frac{1}{2}\ln |\sec(\theta) + \tan(\theta)| \right) + C \\ &= \frac{1}{2} \left( \sqrt{x^{2} + 2x} - \ln \left| x + 1 + \frac{\sqrt{x^{2} + 2x}}{x + 1} \right| \right) + C \end{align*}$$
(d). $\int \frac{x^{2} + 1}{(x^{2} - 2x + 2)^{2}} \; dx$
먼저, 식을 완전제곱식으로 바꾸면 $\frac{x^{2} + 1}{(x^{2} - 2x + 2)^{2}} = \frac{x^{2} + 1}{((x - 1)^{2} + 1)^{2}}$이다. 이제, $x - 1 = u$라고 두면 다음과 같이 적분할 수 있다.
$$\begin{align*} \int \frac{x^{2} + 1}{(x^{2} - 2x + 2)^{2}} \; dx &= \int \frac{x^{2} + 1}{((x - 1)^{2} + 1)^{2}} \; dx \\ &= \int \frac{(u + 1)^{2} + 1}{(u^{2} + 1)^{2}} \; du \\ &= \int \frac{u^{2} + 2u + 2}{(u^{2} + 1)^{2}} \; du \\ &= \int \frac{u^{2} + 2u + 2}{(u^{2} + 1)^{2}} \; du \\ &= \int \left( \frac{u^{2}}{(u^{2} + 1)^{2}} + \frac{2u}{(u^{2} + 1)^{2}} + \frac{2}{(u^{2} + 1)^{2}} \right) \; du \end{align*}$$
1). $\int \frac{u^{2}}{(u^{2} + 1)^{2}} \; du$
$u = \tan(\theta)$라고 하면 $du = \sec^{2}(\theta) d\theta$이다.
$$\begin{align*} \int \frac{u^{2}}{(u^{2} + 1)^{2}} \; du &= \int \frac{\tan^{2}(\theta)}{(\tan^{2}(\theta) + 1)^{2}} \cdot \sec^{2}(\theta) \; d\theta \\ &= \int \frac{\tan^{2}(\theta}{\sec^{2}(\theta)} \; d\theta \\ &= \int \sin^{2}(\theta) \; d\theta \\ &= \int \frac{1 - \cos(2\theta)}{2} \; d\theta \\ &= \frac{1}{2} \left( \theta - \frac{1}{2}\sin(2\theta) \right) + C \\ &= \frac{1}{2} \left( \theta - \sin(\theta)\cos(\theta) \right) + C \\ &= \frac{1}{2} \left( \arctan(u) - \frac{u}{u^{2} + 1} \right) + C \\ &= \frac{1}{2} \left( \arctan(x - 1) - \frac{x - 1}{x^{2} - 2x + 2} \right) + C \end{align*}$$
2). $\int \frac{u}{(u^{2} + 1)^{2}} \; du$
$u^{2} + 1= v$라고 하면 $2u du = dv \rightarrow du = \frac{1}{2u} dv$이다.
$$\begin{align*} \int \frac{u}{(u^{2} + 1)^{2}} \; du &= \int \frac{u}{v^{2}} \cdot \frac{1}{2u} \; dv \\ &= \frac{1}{2} \int \frac{1}{v^{2}} \; dv \\ &= \frac{1}{2} \left( -\frac{1}{v} \right) + C_{2} \\ &= \frac{1}{2} \left( -\frac{1}{u^{2} + 1} \right) + C_{2} \\ &= \frac{1}{2} \left( -\frac{1}{x^{2} - 2x + 2} \right) + C_{2} \end{align*}$$
3). $\int \frac{1}{(u^{2} + 1)^{2}} \; du$
$u = \tan(\theta)$라고 하면 $du = \sec^{2}(\theta) \; d\theta$이다.
$$\begin{align*} \int \frac{1}{(u^{2} + 1)^{2}} \; du &= \int \frac{1}{(\tan^{2}(\theta) + 1)^{2}} \cdot \sec^{2}(\theta) \; d\theta \\ &= \int \frac{1}{\sec^{2}(\theta)} \; d\theta \\ &= \int \cos^{2}(\theta) \; d\theta \\ &= \int \frac{1 + \cos(2\theta)}{2} \; d\theta \\ &= \frac{1}{2} \left( \theta + \frac{1}{2}\sin(2\theta) \right) + C_{3} \\ &= \frac{1}{2} \left( \theta + \sin(\theta)\cos(\theta) \right) + C_{3} \\ &= \frac{1}{2} \left( \arctan(u) + \frac{u}{u^{2} + 1} \right) + C_{3} \\ &= \frac{1}{2} \left( \arctan(x - 1) + \frac{x - 1}{x^{2} - 2x + 2} \right) + C_{3} \end{align*}$$
1), 2), 3)의 적분결과에 의해 기존 적분식은 다음과 같이 풀 수 있다.
$$\begin{align*} \int \frac{x^{2} + 1}{(x^{2} - 2x + 2)^{2}} \; dx &= \int \left( \frac{u^{2}}{(u^{2} + 1)^{2}} + \frac{2u}{(u^{2} + 1)^{2}} + \frac{2}{(u^{2} + 1)^{2}} \right) \; du \\ &= \frac{1}{2} \left( \arctan(x - 1) - \frac{x - 1}{x^{2} - 2x + 2} \right) -\frac{1}{x^{2} - 2x + 2} + \arctan(x - 1) + \frac{x - 1}{x^{2} - 2x + 2} + C \\ &= \frac{3}{2} \arctan(x - 1) + \frac{x - \frac{3}{2}}{2(x^{2} - 2x + 2)} + C \end{align*}$$
(e). $\int x\sqrt{1 - x^{4}} \; dx$
$x = \sqrt{\sin(\theta)}$라고 하면 $dx = \frac{\cos(\theta)}{2\sqrt{\sin(\theta)}} \; d\theta$이다.
$$\begin{align*} \int x\sqrt{1 - x^{4}} \; dx &= \int \sqrt{\sin(\theta)} \sqrt{1 - \sin^{2}(\theta)} \cdot \frac{\cos(\theta)}{2\sqrt{\sin(\theta)}} \; d\theta \\ &= \frac{1}{2} \int \cos^{2}(\theta) \; d\theta \\ &= \frac{1}{2} \int \frac{1 + \cos(2\theta)}{2} \; d\theta \\ &= \frac{1}{4} \int \left( \theta + \frac{1}{2}\sin(2\theta) \right) \; d\theta \\ &= \frac{1}{4} \left( \theta + \sin(\theta)\cos(\theta) \right) + C \\ &= \frac{1}{4} \left( \arcsin(x^{2}) - x^{2}\sqrt{1 - x^{2}} \right) \end{align*}$$
연습문제5. 두 적분을 증명하라.
(a). 삼각함수 치환법을 통해 $\int \frac{1}{\sqrt{x^{2} + a^{2}}} \; dx = \ln (x + \sqrt{x^{2} + a^{2}}) + C$임을 증명하라.
(b). 쌍곡함수 치환법을 통해 $\int \frac{1}{\sqrt{x^{2} + a^{2}}} \; dx = \arcsinh \left( \frac{a}{x} \right) + C$임을 증명하라.
(a). 삼각함수 치환법을 통해 $\int \frac{1}{\sqrt{x^{2} + a^{2}}} \; dx = \ln (x + \sqrt{x^{2} + a^{2}}) + C$임을 증명하라.
$x = a\tan(\theta)$라고 하면 $dx = a\sec^{2}(\theta) \; d\theta$이다.
$$\begin{align*} \int \frac{1}{\sqrt{x^{2} + a^{2}}} \; dx &= \int \frac{1}{\sqrt{a^{2}\tan^{2}(\theta) + a^{2}}} \cdot (a\sec^{2}(\theta)) \; d\theta \\ &= \int \sec(\theta) \; d\theta \\ &= \ln (\sec(\theta) + \tan(\theta)) + C \\ &= \ln (x + \sqrt{x^{2} + a^{2}}) + C \end{align*}$$
(b). 쌍곡함수 치환법을 통해 $\int \frac{1}{\sqrt{x^{2} + a^{2}}} \; dx = \arcsinh \left( \frac{a}{x} \right) + C$임을 증명하라.
$x = a\sinh(\theta)$라고 하면 $dx = a\cosh(\theta) \; d\theta$이다.
$$\begin{align*} \int \frac{1}{\sqrt{x^{2} + a^{2}}} \; dx &= \int \frac{1}{\sqrt{a^{2}\sinh^{2}(\theta) + a^{2}}} \cdot (a\cosh(\theta)) \; d\theta \\ &= \int 1 \; d\theta \\ &= \theta + C \\ &= \arcsinh \left( \frac{x}{a} \right) + C \end{align*}$$
연습문제6. 주어진 영역의 넓이를 구하여라. 여기서, 부채꼴의 반지름은 $r$이고 각은 $\theta$이다.
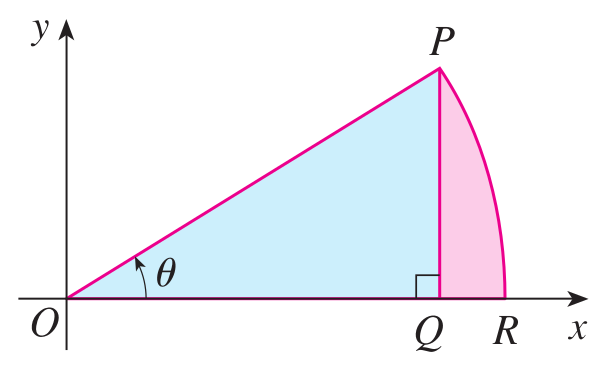
주어진 영역의 넓이는 $\triangle OPQ$와 호 $PQR$의 넓이의 합이다.
1). $\triangle OPQ$의 넓이를 A라고 할 때 삼각형이므로 $A = \frac{(r\cos(theta) \cdot (r\sin(\theta))}{2} = \frac{r^{2}}{2}(\sin(\theta)\cos(\theta))$이다.
2). 호 $PQR$의 넓이를 $B$라고 할 때 해당 영역은 원 $x^{2} + y^{2} = r^{2}$에서 구간 $[r\cos(\theta), r]$에서의 밑넓이와 동일하므로 다음과 같이 적분할 수 있다.
$$\begin{align*} B &= \int_{r\cos(\theta)}^{r} \sqrt{r^{2} - x^{2}} \; dx \end{align*}$$
이를 위해 $x = r\cos(\phi)$라고 하면 $dx = -r\sin(\phi) d\phi$이다.
$$\begin{align*} \int \sqrt{r^{2} - x^{2}} \; dx &= \int \sqrt{r^{2} - r^{2}\cos^{2}(\phi)} \cdot (-r\sin(\phi)) \; d\theta \\ &= \int -r^{2}\sin^{2}(\phi) \; d\phi \\ &= -r^{2} \int \frac{1 - \cos(2\phi)}{2} \; d\phi \\ &= -\frac{r^{2}}{2} \left( \phi - \frac{1}{2}\sin(2\phi) \right) + C \\ &= -\frac{r^{2}}{2} \left(\phi - \sin(\phi)\cos(\phi) \right) + C \\ &= -\frac{r^{2}}{2} \left( \arccos(\frac{x}{r}) - \frac{x\sqrt{r^{2} - x^{2}}}{r^{2}} \right) + C \\ &= \frac{r^{2}}{2} \left( \frac{x\sqrt{r^{2} - x^{2}}}{r^{2}} - \arccos(\frac{x}{r}) \right)\end{align*}$$
따라서 다음과 같이 정적분을 계산할 수 있다.
$$\begin{align*} B &= \int_{r\cos(\theta)}^{r} \sqrt{r^{2} - x^{2}} \; dx \\ &= \frac{r^{2}}{2} \left[ \frac{x\sqrt{r^{2} - x^{2}}}{r^{2}} - \arccos(\frac{r}{x}) \right]_{r\cos(\theta)}^{r} \\ &= \frac{r^{2}}{2} \left[ \left( 0 - \arccos(1) \right) - \left( \frac{r^{2}\cos(\theta)\sin(\theta)}{r^{2}} - \arccos(\cos(\theta)) \right) \right] \\ &= -\frac{r^{2}}{2} \sin(\theta)\cos(\theta) + \frac{r^{2}}{2}\theta \end{align*}$$
이제, 1), 2)의 적분결과를 합치면 된다.
$$A + B = \left( \frac{r^{2}}{2} \sin(\theta)\cos(\theta) \right) + \left( -\frac{r^{2}}{2}\sin(\theta)\cos(\theta) + \frac{r^{2}}{2} \theta \right) = \frac{r^{2}}{2} \theta $$
참고자료 및 그림출처
Calculus(J. Stewart)
'수학 > 미적분학' 카테고리의 다른 글
| 미적분학 - 적분 전략 (0) | 2022.03.24 |
|---|---|
| 미적분학 - 부분분수를 활용한 유리함수 적분 (0) | 2022.03.22 |
| 미적분학 - 복잡한 삼각함수 적분 (0) | 2022.03.16 |
| 미적분학 - 부분적분 (0) | 2022.03.14 |
| 미적분학 - 함수의 평균 (0) | 2022.03.12 |
안녕하세요. 지난 포스팅의 미적분학 - 복잡한 삼각함수 적분 2에서는 $\int \tan^{m}(x) \sec^{n}(x) \; dx$ 꼴의 적분을 몇몇 케이스와 예제로 나누어 적분을 해보았습니다. 이전과 마찬가지로 핵심은 삼각함수와 관련된 항등식을 적절하게 활용하는 것이였습니다. 오늘은 치환적분을 통해서 삼각함수를 적분해야하는 경우에 대해서 설명드리도록 하겠습니다.
간단한 예제로 $\int \frac{\sqrt{9 - x^{2}}}{x^{2}} \; dx$를 구해보도록 하겠습니다. 언뜻보면 삼각함수와 관련없어 보이지만 $x = 3\sin(\theta)$라고 두면 $\sqrt{9 - x^{2}} = \sqrt{9 - 9\sin^{2}(\theta)} = 3\sqrt{1 - \sin^{2}(\theta)} = 3 \left|\cos(\theta)\right| = 3\cos(\theta)$가 됩니다. 또한, $dx = 3\cos(\theta) d\theta$입니다. 따라서 아래와 같이 적분 가능합니다.
$$\begin{align*} \int \frac{\sqrt{9 - x^{2}}}{x^{2}} &= \int \frac{3\cos(\theta)}{9\sin^{2}(\theta)}3\cos(\theta) \theta \\ &= \int \frac{\cos^{2}(\theta)}{\sin^{2}(\theta)} \; d\theta \\ &= \int \cot^{2}(\theta) \; d\theta \\ &= \int \left(\csc^{2}(\theta) - 1\right) \; d\theta \\ &= -\cot(\theta) - \theta + C\end{align*}$$
이때, $\cot(\theta) = \frac{\sqrt{9 - x^{2}}}{x}$이고 $\sin(\theta) = \frac{x}{3} \Rightarrow \theta = \arcsin(\frac{x}{3})$ 이기 때문에 최종적으로 아래의 결과를 얻을 수 있습니다.
$$\begin{align*} \int \frac{\sqrt{9 - x^{2}}}{x^{2}} &= -\cot(\theta) - \theta + C \\ &= \frac{\sqrt{9 - x^{2}}}{x} - \arcsin(\frac{x}{3}) + C\end{align*}$$
다음 예제로 $\int \frac{1}{x^{2}\sqrt{x^{2} + 4}} \; dx$를 구해보도록 하겠습니다. 이 역시 언뜻보면 삼각함수와 큰 관련은 없어보입니다. 하지만, $x = 2\tan(\theta) \Rightarrow dx = \sec^{2}(\theta) d\theta$를 얻을 수 있습니다. 이제 식을 변형해보도록 하겠습니다.
$$\frac{1}{x^{2}\sqrt{x^{2} + 4}} = \frac{1}{4\tan^{2}(\theta)\sqrt{4\tan^{2}(\theta) + 4}} = \frac{1}{8\tan^{2}(\theta) \sec(\theta)}$$
따라서 아래와 같이 적분할 수 있습니다.
$$\begin{align*} \int \frac{1}{x^{2}\sqrt{x^{2} + 4}} \; dx &= \int \frac{1}{8\tan^{2}(\theta)\sec(\theta)} \; (2\sec^{2}(\theta) du) \\ &= \frac{1}{4} \int \frac{\sec(\theta)}{\tan^{2}(\theta)} \; d\theta\end{align*}$$
저희는 여기서 추가적으로 식을 변형할 수 있습니다.
$$\frac{\sec(\theta)}{\tan^{2}(\theta)} = \frac{1}{\cos(\theta)} \cdot \frac{\cos^{2}(\theta)}{\sin^{2}(\theta)} = \frac{\cos(\theta)}{\sin^{2}(\theta)}$$
그러므로 다시 적분식을 정리하도록 하겠습니다.
$$\begin{align*} \int \frac{1}{x^{2}\sqrt{x^{2} + 4}} \; dx &= \frac{1}{4} \int \frac{\sec(\theta)}{\tan^{2}(\theta)} \; d\theta \\ &= \frac{1}{4} \int \frac{\cos(\theta)}{\sin^{2}(\theta)} \; d\theta \end{align*}$$
이제, 저희는 $\sin(\theta) = u$라고 하면 $\cos(\theta) d\theta = du \Rightarrow d\theta = \frac{1}{\cos(\theta)} du$를 얻을 수 있습니다. 그러면 다시 적분을 진행해보도록 하겠습니다.
$$\begin{align*} \int \frac{1}{x^{2}\sqrt{x^{2} + 4}} \; dx &= \frac{1}{4} \int \frac{\cos(\theta)}{\sin^{2}(\theta)} \; d\theta \\ &= \frac{1}{4} \int \frac{\cos(\theta)}{\sin^{2}(\theta)} \\ &= \frac{1}{4} \int \frac{\cos(\theta)}{u^{2}} \; \left(\frac{1}{\cos(\theta)} du\right) = \frac{1}{4} \int \frac{1}{u^{2}} \; du \\ &= \frac{1}{4} \left(-\frac{1}{u}\right) + C \\ &= -\frac{1}{4\sin(\theta)} + C\end{align*}$$
마지막으로 저희가 $x = 2\tan(\theta)$로 치환했기 때문에 $\sin(\theta) = \frac{x}{\sqrt{x^{2} + 4}}$를 얻을 수 있습니다. 따라서, 마지막 식은 아래와 같습니다.
$$\begin{align*} \int \frac{1}{x^{2}\sqrt{x^{2} + 4}} \; dx &= -\frac{1}{4\sin(\theta)} + C \\ &= -\frac{\sqrt{x^{2} + 4}}{4x} + C \end{align*}$$
연습문제1. 주어진 적분을 계산하라.
(a). $\int_{0}^{2\sqrt{3}} \frac{x}{\sqrt{16 - x^{2}}} \; dx$
(b). $\int_{\sqrt{2}}^{2} \frac{1}{x^{3}\sqrt{x^{2} - 1}} \; dx$
(c). $\int_{1}^{2} \frac{\sqrt{x^{2} - 1}}{x} \; dx$
(d). $\int \frac{1}{x^{2}\sqrt{25 - x^{2}}} \; dx$
(e). $\int \frac{x^{3}}{\sqrt{x^{2} + 100}} \; dx$
(f). $\int \frac{1}{\sqrt{x^{2} + 16}} \; dx$
(g). $\int \frac{x^{5}}{\sqrt{x^{2} + 2}} \; dx$
(a). $\int_{0}^{2\sqrt{3}} \frac{x}{\sqrt{16 - x^{2}}} \; dx$
$x = 4\sin(\theta)$라고 하면 $dx = 4\cos(\theta) d\theta$이고 적분구간은 $[0, 2\sqrt{3}] \xrightarrow{\theta = \arcsin(\frac{x}{4})} [\arcsin(0), \arcsin(\frac{\sqrt{3}}{2})] = [0, \frac{\pi}{3}]$이다.
$$\begin{align*} \int_{0}^{2\sqrt{3}} \frac{x}{\sqrt{16 - x^{2}}} \; dx &= \int_{0}^{\frac{\pi}{3}} \frac{4sin(\theta)}{\sqrt{16 - 16\sin^{2}(\theta)}} \cdot 4\cos(\theta) d\theta \\ &= \int_{0}^{\frac{\pi}{3}} \frac{16\sin(\theta)\cos(\theta)}{4\cos(\theta)} \; d\theta \\ &= \int_{0}^{\frac{\pi}{3}} 4\sin(\theta) \; d\theta \\ &= [-4\cos(\theta)]_{0}^{\frac{\pi}{3}} \\ &= -4(\cos(\frac{\pi}{3}) - \cos(0)) \\ &= -4(\frac{1}{2} - 1) = 2 \end{align*}$$
(b). $\int_{\sqrt{2}}^{2} \frac{1}{x^{3}\sqrt{x^{2} - 1}} \; dx$
$x = \sec(\theta)$라고 하면 $dx = \sec(\theta)\tan(\theta) d\theta$이고 적분구간은 $[\sqrt{2}, 2] \xrightarrow{\theta = \arcsec(x)} [\arcsin(\frac{1}{\sqrt{2}}), \arcsin(\frac{1}{2})] = [\frac{\pi}{4}, \frac{\pi}{3}]$이다.
$$\begin{align*} \int_{\sqrt{2}}^{2} \frac{1}{x^{3}\sqrt{x^{2} - 1}} \; dx &= \int_{\frac{\pi}{4}}^{\frac{\pi}{3}} \frac{1}{\sec^{3}(\theta) \sqrt{\sec^{2}(\theta) - 1}} \cdot \sec(\theta)\tan(\theta) d\theta \\ &= \int_{\frac{\pi}{4}}^{\frac{\pi}{3}} \frac{\sec(\theta)\tan(\theta)}{\sec^{3}(\theta)\tan(\theta)} \; d\theta \\ &= \int_{\frac{\pi}{4}}^{\frac{\pi}{3}} \cos^{2}(\theta) \; d\theta \\ &= \int_{\frac{\pi}{4}}^{\frac{\pi}{3}} \left( \frac{1 + 2\cos(2\theta)}{2} \right) \; d\theta \\ &= \frac{1}{2}\int_{\frac{\pi}{4}}^{\frac{\pi}{3}} (1 + 2\cos(2\theta)) \; d\theta \\ &= \frac{1}{2} \left[ \theta + \sin(2\theta) \right]_{\frac{\pi}{4}}^{\frac{\pi}{3}} \\ &= \frac{1}{2} \left[ \left( \frac{\pi}{3} + \sin(\frac{2\pi}{3}) \right) - \left( \frac{\pi}{4} + \sin(\frac{\pi}{2}) \right) \right] = \frac{1}{2} \left( \frac{\pi}{12} + \frac{\sqrt{3}}{2} - 1 \right)\end{align*}$$
(c). $\int_{1}^{2} \frac{\sqrt{x^{2} - 1}}{x} \; dx$
$x = \sec(\theta)$라고 하면 $dx = \sec(\theta)\tan(\theta) d\theta$이고 적분구간은 $[1, 2] \xrightarrow{\theta = \arcsec(x)} [\arcsin(1), \arcsin(\frac{1}{2})] = [0, \frac{\pi}{3}]$이다.
$$\begin{align*} \int_{1}^{2} \frac{x^{2} - 1}{x} \; dx &= \int_{0}^{\frac{\pi}{3}} \frac{\sqrt{\sec^{2}(\theta) - 1}}{\sec(\theta)} \cdot (\sec(\theta)\tan(\theta)) \; d\theta \\ &= \int_{0}^{\frac{\pi}{3}} \frac{\tan(\theta)}{\sec(\theta)} \cdot (\sec(\theta)\tan(\theta)) \; d\theta \\ &= \int_{0}^{\frac{\pi}{3}} \tan^{2}(\theta) \; d\theta \\ &= \int_{0}^{\frac{\pi}{3}} (\sec^{2}(\theta) - 1) \; d\theta \\ &= \left[ \tan(\theta) - \theta \right]_{0}^{\frac{\pi}{3}} \\ &= \left( \tan(\frac{\pi}{3}) - \frac{\pi}{3} \right) - \left( \tan(0) - 0 \right) = \sqrt{3} - \frac{\pi}{3} \end{align*}$$
(d). $\int \frac{1}{x^{2}\sqrt{25 - x^{2}}} \; dx$
$x = 5\sin(\theta)$라고 하면 $dx = 5\cos(\theta) d\theta$이다.
$$\begin{align*} \int \frac{1}{x^{2}\sqrt{25 - x^{2}}} \; dx &= \int \frac{1}{25\sin^{2}(\theta)\sqrt{25 - 25\sin^{2}(\theta)}} \cdot (5\cos(\theta)) \; d\theta \\ &= \int \frac{1}{25\sin^{2}(\theta)} \; d\theta \\ &= \frac{1}{25} \int \csc^{2}(\theta) \; d\theta \\ &= -\frac{1}{25} \cot(\theta) + C \\ &= -\frac{1}{25} \frac{\sqrt{25 - x^{2}}}{x} + C \end{align*}$$
(e). $\int \frac{x^{3}}{\sqrt{x^{2} + 100}} \; dx$
$x = 10\tan(\theta)$라고 하면 $dx = 10\sec^{2}(\theta) d\theta$이다.
$$\begin{align*} \int \frac{x^{3}}{\sqrt{x^{2} + 100}} \; dx &= \int \frac{1000\tan^{3}(\theta)}{\sqrt{100\tan^{2}(\theta) + 1}} \cdot (10\sec^{2}(\theta)) \; d\theta \\ &= 1000\int \tan^{3}(\theta)\sec(\theta) \; d\theta \\ &= 1000 \int \tan^{2}(\theta) \tan(\theta)\sec(\theta) \; d\theta \\ &= 1000 \int (\sec^{2}(\theta) - 1) \tan(\theta)\sec(\theta) \; d\theta \end{align*}$$
여기서 $\sec(\theta) = u$라고 하면 $\sec(\theta)\tan(\theta) d\theta = du \rightarrow d\theta = \frac{1}{\sec(\theta)\tan(\theta)} du$이다.
$$\begin{align*} \int \frac{x^{3}}{\sqrt{x^{2} + 100}} \; dx &= 1000 \int (\sec^{2}(\theta) - 1) \tan(\theta)\sec(\theta) \; d\theta \\ &= 1000 \int (u^{2} - 1) \tan(\theta)\sec(\theta) \cdot \frac{1}{\sec(\theta)\tan(\theta)} \; d\theta \\ &= 1000 \int (u^{2} - 1) \; du \\ &= 1000 \left( \frac{1}{3}u^{3} - u \right) + C \\ &= 1000 \left( \frac{1}{3}\sec^{3}(\theta) - \sec(\theta) \right) + C \\ &= 1000 \left( \frac{(100 + x^{2})\sqrt{100 + x^{2}}}{3000} - \frac{\sqrt{100 + x^{2}}}{10} \right) + C \end{align*}$$
(f). $\int \frac{1}{\sqrt{x^{2} + 16}} \; dx$
$x = 4\tan(\theta)$라고 하면 $dx = 4\sec^{2}(\theta) d\theta$이다.
$$\begin{align*} \int \frac{1}{\sqrt{x^{2} + 16}} \; dx &= \int \frac{1}{\sqrt{16\tan^{2}(\theta) + 16}} \cdot (4\sec^{2}(\theta)) \; d\theta \\ &= \int \sec(\theta) \; d\theta \\ &= \ln|\sec(\theta) + \tan(\theta)| + C \\ &= \ln \left| \frac{\sqrt{16 + x^{2}}}{4} + \frac{x}{4} \right| + C \end{align*}$$
(g). $\int \frac{x^{5}}{\sqrt{x^{2} + 2}} \; dx$
$x = \sqrt{2}\tan(\theta)$라고 하면 $dx = \sqrt{2}\sec^{2}(\theta) d\theta$이다.
$$\begin{align*} \int \frac{x^{5}}{\sqrt{x^{2} + 2}} \; dx &= \int \frac{4\sqrt{2}\tan^{5}(\theta)}{\sqrt{2} \sec(\theta)} \cdot (\sqrt{2} \sec^{2}(\theta)) \; d\theta \\ &= 4\sqrt{2} \int \tan^{5}(\theta)\sec(\theta) \; d\theta \\ &= 4\sqrt{2} \int \tan^{4}(\theta) \tan(\theta)\sec(\theta) \; d\theta \\ &= 4\sqrt{2} \int (\sec^{2}(\theta) - 1)^{2} \tan(\theta)\sec(\theta) \; d\theta \end{align*}$$
여기서 $\sec(\theta) = u$라고 하면 $\sec(\theta)\tan(\theta) d\theta = du \rightarrow d\theta = \frac{1}{\sec(\theta)\tan(\theta)} du$이다.
$$\begin{align*} \int \frac{x^{5}}{\sqrt{x^{2} + 2}} \; dx &= 4\sqrt{2} \int (\sec^{2}(\theta) - 1)^{2} \tan(\theta)\sec(\theta) \; d\theta \\ &= 4\sqrt{2} \int (u^{2} - 1)^{2} \; du \\ &= 4\sqrt{2} \int (u^{4} - 2u^{2} + 1) \; du \\ &= 4\sqrt{2} \left( \frac{1}{5}u^{5} - \frac{2}{3}u^{3} + u \right) + C \\ &= 4\sqrt{2} \left( \frac{1}{5}\sec^{5}(\theta) - \frac{2}{3}\sec^{3}(\theta) + \sec(\theta) \right) + C \\ &= 4\sqrt{2} \left( \frac{(x^{2} + 2)^{2}\sqrt{x^{2} + 2}}{4\sqrt{2}} - \frac{2}{3} \frac{(x^{2} + 2)\sqrt{x^{2} + 2}}{2} + \frac{\sqrt{x^{2} + 2}}{\sqrt{2}} \right) + C \end{align*}$$
연습문제2. 주어진 적분을 계산하라.
(a). $\int \sqrt{1 - 4x^{2}} \; dx$
(b). $\int_{0}^{1} x\sqrt{x^{2} + 4} \; dx$
(c). $\int \frac{\sqrt{x^{2} - 9}}{x^{3}} \; dx$
(d). $\int \frac{1}{x\sqrt{5 - x^{2}}} \; dx$
(e). $\int_{0}^{a} x^{2}\sqrt{a^{2} - x^{2}} \; dx$
(a). $\int \sqrt{1 - 4x^{2}} \; dx$
$x = \frac{1}{2}\sin(\theta)$라고 하면 $dx = \frac{1}{2}\cos(\theta) d\theta$이다.
$$\begin{align*} \int \sqrt{1 - 4x^{2}} \; dx &= \int \sqrt{1 - \sin^{2}(\theta)} \cdot (\frac{1}{2}\cos(\theta)) \; d\theta \\ &= \frac{1}{2} \int \cos^{2}(\theta) \; d\theta \\ &= \frac{1}{4} \int \left( 1 + \cos(2\theta) \right) \; d\theta \\ &= \frac{1}{4} \left( \theta + \frac{1}{2} \sin(2\theta) \right) + C \\ &= \frac{1}{4} (\arcsin(2x) + \frac{1}{2} (\sqrt{1 - 4x^{2}} - 4x^{2}) ) + C \end{align*}$$
(b). $\int_{0}^{1} x\sqrt{x^{2} + 4} \; dx$
$x = 2\tan(\theta)$라고 하면 $dx = 2\sec^{2}(\theta) d\theta$이고적분구간은 $[0, 1] \xrightarrow{\theta = \arctan(\frac{x}{2})} [\arctan(0), \arctan(\frac{1}{2})] = [0, \alpha]$이다. 여기서, $\alpha = \arctan(\frac{1}{2})$이다.
$$\begin{align*} \int_{0}^{1} x\sqrt{x^{2} + 4} \; dx &= \int_{0}^{\alpha} 2\tan(\theta) \sqrt{4\tan^{2}(\theta) + 4} \cdot (2\sec^{2}(\theta)) \; d\theta \\ &= 8\int_{0}^{\alpha} \tan(\theta) \sec^{3}(\theta) \; d\theta \end{align*}$$
여기서, $\sec(\theta) = u$라고 하면 $\sec(\theta) \tan(\theta) d\theta = du \rightarrow d\theta = \frac{1}{\sec(\theta)\tan(\theta)} du$이고 적분구간은 $[0, \alpha] \xrightarrow{u = \sec(\theta)} [\sec(0), \sec(\alpha)] = [1, \sec(\alpha)]$이다.
$$\begin{align*} \int_{0}^{1} x\sqrt{x^{2} + 4} \; dx &= 8\int_{0}^{\alpha} \tan(\theta) \sec^{3}(\theta) \; d\theta \\ &= 8\int_{1}^{\sec(\alpha)} u^{2} \sec(\theta)\tan(\theta) \cdot \frac{1}{\sec(\theta)\tan(\theta)} \; du \\ &= 8\int_{1}^{\sec(\alpha)} u^{2} \; du \\ &= \left[ \frac{1}{3}u^{3} \right]_{1}^{\sec(\alpha)} = \frac{8}{3} \left(\sec^{3}(\alpha) - 1\right) \end{align*}$$
(c). $\int \frac{\sqrt{x^{2} - 9}}{x^{3}} \; dx$
$x = 3\sec(\theta)$라고 하면 $dx = 3\sec(\theta)\tan(\theta) d\theta$이다.
$$\begin{align*} \int \frac{\sqrt{x^{2} - 9}}{x^{3}} \; dx &= \int \frac{\sqrt{9\sec^{2}(\theta) - 9}}{27\sec^{3}(\theta)} \cdot (3\sec(\theta)\tan(\theta)) \; d\theta \\ &= \int \frac{3\tan(\theta)}{27\sec^{3}(\theta)} \cdot (3\sec(\theta)\tan(\theta)) \; d\theta \\ &= \frac{1}{3} \int \frac{\tan^{2}(\theta)}{\sec^{2}(\theta)} \; d\theta \\ &= \frac{1}{3} \int \sin^{2}(\theta) \; d\theta \\ &= \frac{1}{6} \int (1 - \cos(2\theta)) \; d\theta \\ &= \frac{1}{6} \left( \theta - \frac{1}{2}\sin(2\theta) \right) + C \\ &= \frac{1}{6} \left( \arcsec(\frac{x}{3}) - \frac{6\sqrt{x^{2} - 9}}{x} \right) + C \end{align*}$$
(d). $\int \frac{1}{x\sqrt{5 - x^{2}}} \; dx$
$x = \sqrt{5}\sin(\theta)$라고 하면 $dx = \sqrt{5}\cos(\theta) d\theta$이다.
$$\begin{align*} \int \frac{1}{x\sqrt{5 - x^{2}}} \; dx &= \int \frac{1}{\sqrt{5}\sin(\theta) \sqrt{5 - 5\sin^{2}(\theta)}} \cdot (\sqrt{5}\cos(\theta)) \; d\theta \\ &= \frac{1}{\sqrt{5}} \int \frac{1}{\sin(\theta)} \; d\theta \\ &= \frac{1}{\sqrt{5}} \ln \left| \frac{1}{\csc(\theta) + \cot(\theta)} \right| + C \\ &= \frac{1}{\sqrt{5}} \ln \left| \frac{x}{\sqrt{5 - x^{2}} + \sqrt{5}} \right| + C \end{align*}$$
(e). $\int_{0}^{a} x^{2}\sqrt{a^{2} - x^{2}} \; dx$
$x = a\sin(\theta)$라고 하면 $dx = a\cos(\theta) d\theta$이고 적분구간은 $[0, a] \xrightarrow{\theta = \arcsin(\frac{x}{a})} [\arcsin(0), \arcsin(1)] = [0, \frac{\pi}{2}] $이다.
$$\begin{align*} \int_{0}^{a} x^{2}\sqrt{a^{2} - x^{2}} \; dx &= \int_{0}^{\frac{\pi}{2}} a^{2}\sin^{2}(\theta) \sqrt{a^{2} - a^{2}\sin^{2}(\theta)} \cdot (a\cos(\theta))\; d\theta \\ &= a^{4} \int_{0}^{\frac{\pi}{2}} (\sin(\theta) \cos(\theta))^{2} \; d\theta \\ &= \frac{a^{4}}{4} \int_{0}^{\frac{\pi}{2}} \sin^{2}(2\theta) \; d\theta \\ &= \frac{a^{4}}{8} \int_{0}^{\frac{\pi}{2}} (1 - \cos(4\theta)) \; d\theta \\ &= \frac{a^{4}}{8} \left[ \theta - \frac{1}{4}\sin(4\theta) \right]_{0}^{\frac{\pi}{2}} = \frac{a^{4}\pi}{16} \end{align*}$$
연습문제3. 주어진 적분을 계산하라.
(a). $\int \frac{x}{\sqrt{x^{2} - 7}} \; dx$
(b). $\int \frac{\sqrt{1 + x^{2}}}{x} \; dx$
(c). $\int \frac{x}{\sqrt{25 - x^{2}}} \; dx$
(d). $\int_{0}^{1} \sqrt{x^{2} + 1} \; dx$
(e). $\int \sqrt{5 + 4x - x^{2}} \; dx$
(a). $\int \frac{x}{x^{2} - 7} \; dx$
$x = \sqrt{7}\sec(\theta)$라고 하면 $dx = \sqrt{7}\sec(\theta)\tan(\theta) d\theta$이다.
$$\begin{align*} \int \frac{x}{\sqrt{x^{2} - 7}} \; dx &= \int \frac{\sqrt{7}\sec(\theta)}{\sqrt{7\sec^{2}(\theta) - 7}} \cdot (\sqrt{7}\sec(\theta)\tan(\theta)) \; d\theta \\ &= \int \sqrt{7}\sec^{2}(\theta) \; d\theta \\ &= \sqrt{7}\tan(\theta) + C \\ &= \sqrt{7} \cdot \frac{\sqrt{x^{2} - 7}}{\sqrt{7}} + C \\ &= \sqrt{x^{2} - 7} + C \end{align*}$$
(b). $\int \frac{\sqrt{1 + x^{2}}}{x} \; dx$
$x = \tan(\theta)$라고 하면 $dx = \sec^{2}(\theta) d\theta$이다.
$$\begin{align*} \int \frac{\sqrt{1 + x^{2}}}{x} \; dx &= \int \frac{\sqrt{1 + \tan^{2}(\theta)}}{\tan(\theta)} \cdot (\sec^{2}(\theta)) \; d\theta \\ &= \int \frac{\sec^{3}(\theta)}{\tan(\theta)} \; d\theta \\ &= \int \frac{(1 + \tan^{2}(\theta))\sec(\theta)}{\tan(\theta)} \; d\theta \\ &= \int \left( \frac{\sec(\theta)}{\tan(\theta)} + \sec(\theta)\tan(\theta) \right) \; d\theta \\ &= \int \left( \csc(\theta) + \tan(\theta)\sec(\theta) \right) \; d\theta \\ &= \ln \left|\frac{1}{\csc(\theta) + \cot(\theta)}\right| + \sec(\theta) + C \\ &= \ln \left| \frac{1}{\sqrt{x^{2} + 1} + \frac{1}{x}} \right| + \frac{\sqrt{x^{2} + 1}}{x} + C \end{align*}$$
(c). $\int \frac{x}{\sqrt{25 - x^{2}}} \; dx$
$x = 5\sin(\theta)$라고 하면 $dx = 5\cos(\theta) d\theta$이다.
$$\begin{align*} \int \frac{x}{\sqrt{25 - x^{2}}} \; dx &= \int \frac{5\sin(\theta)}{\sqrt{25 - 25\sin^{2}(\theta)}} \cdot (5\cos(\theta)) \; d\theta \\ &= \int \frac{5\sin(\theta)}{5\cos(\theta)} \cdot (5\cos(\theta)) \; d\theta \\ &= 5\int \sin(\theta) \; d\theta \\ &= -5\cos(\theta) + C \\ &= -5 \cdot \frac{\sqrt{25 - x^{2}}}{5} = C \\ &= -\sqrt{25 - x^{2}} + C \end{align*}$$
(d). $\int_{0}^{1} \sqrt{x^{2} + 1} \; dx$
$x = \tan(\theta)$라고 하면 $dx = \sec^{2}(\theta) d\theta$이고 적분구간은 $[0, 1] \xrightarrow{\theta = \arctan(x)} [\arctan(0), \arctan(1)] = [0, \frac{\pi}{4}] $이다.
$$\begin{align*} \int_{0}^{1} \sqrt{x^{2} + 1} \; dx &= \int_{0}^{\frac{\pi}{4}} \sqrt{\tan^{2}(\theta) + 1} \cdot (\sec^{2}(\theta)) \; d\theta \\ &= \int_{0}^{\frac{\pi}{4}} \sec^{3}(\theta) \; d\theta \\ &= \int_{0}^{\frac{\pi}{4}} (1 + \tan^{2}(\theta)) \sec(\theta) \; d\theta \\ &= \int_{0}^{\frac{\pi}{4}} \left( \sec(\theta) + \tan^{2}(\theta)\sec(\theta) \right) \; d\theta \end{align*}$$
1). $\int_{0}^{\frac{\pi}{4}} \sec(\theta) \; d\theta = \left[ \ln|\sec(\theta) + \tan(\theta)| \right]_{0}^{\frac{\pi}{4}} = \ln (\sqrt{2} + 1)$
2). $f = \tan(\theta)$ 그리고 $g^{'} = \tan(\theta)\sec(\theta)$라고 하면 $f^{'} = \sec^{2}(\theta)$ 이고 $g = \sec(\theta)$이다.
$$\begin{align*} \int_{0}^{\frac{\pi}{4}} \tan^{2}(\theta)\sec(\theta) \; d\theta &= \left[ \tan(\theta)\sec(\theta) \right]_{0}^{\frac{\pi}{4}} - \int_{0}^{\frac{\pi}{4}} \sec^{3}(\theta) \; d\theta \\ &= \sqrt{2} - \int_{0}^{\frac{\pi}{4}} \sec^{3}(\theta) \; d\theta \end{align*}$$
이제 식을 정리하면 다음과 같다.
$$\begin{align*} \int_{0}^{1} \sqrt{x^{2} + 1} \; dx &= \int_{0}^{\frac{\pi}{4}} \left( \sec(\theta) + \tan^{2}(\theta)\sec(\theta) \right) \; d\theta \\ &= \ln (\sqrt{2} + 1) + \sqrt{2} - \int_{0}^{\frac{\pi}{4}} \sec^{3}(\theta) \; d\theta \\ \Rightarrow& \int_{0}^{1} \sqrt{x^{2} + 1} \; dx = \int_{0}^{\frac{\pi}{4}} \sec^{3}(\theta) \; d\theta = \frac{1}{2} \left( \ln (\sqrt{2} + 1) + \sqrt{2} \right) \end{align*}$$
(e). $\int \sqrt{5 + 4x - x^{2}} \; dx$
먼저, 식을 완전제곱식으로 바꾸면 $5 + 4x - x^{2} = 9 - (x^{2} - 4x + 4) = 9 - (x - 2)^{2}$이다. 이제, $x - 2 = u$라고 두면 다음과 같이 적분할 수 있다.
$$\begin{align*} \int \sqrt{5 + 4x - x^{2}} \; dx &= \int \sqrt{9 - (x - 2)^{2}} \; dx \\ &= \int \sqrt{9 - u^{2}} \; du \end{align*}$$
이제, $u = 3\sin(\theta)$라고 두면 $du = 3\cos(\theta)$이다.
$$\begin{align*} \int \sqrt{5 + 4x - x^{2}} \; dx &= \int \sqrt{9 - u^{2}} \; du \\ &= \int \sqrt{9 - 9\sin^{2}(\theta)} \cdot (3\cos(\theta)) \; d\theta \\ &= \int 9\cos^{2}(\theta) \; d\theta \\ &= \frac{9}{2} \int \left( 1 + \cos(2\theta) \right) \; d\theta \\ &= \frac{9}{2} \left( \theta + \frac{1}{2}\sin(2\theta) \right) + C \\ &= \frac{9}{2} \left( \arcsin \left( \frac{u}{3} \right) + \frac{1}{2} \frac{u\sqrt{9 - u^{2}}}{9} \right) + C \\ &= \frac{9}{2} \left( \arcsin \left( \frac{1}{3}(x - 2) \right) + \frac{(x - 2)\sqrt{5 + 4x - x^{2}}}{18} \right) + C \end{align*}$$
연습문제4. 주어진 적분을 계산하라.
(a). $\int \frac{x}{\sqrt{x^{2} + x + 1}} \; dx$
(b). $\int \frac{x^{2}}{(3 + 4x - 4x^{2})^{\frac{3}{2}}} \; dx$
(c). $\int \sqrt{x^{2} + 2x} \; dx$
(d). $\int \frac{x^{2} + 1}{(x^{2} - 2x + 2)^{2}} \; dx$
(e). $\int x\sqrt{1 - x^{4}} \; dx$
(a). $\int \frac{x}{\sqrt{x^{2} + x + 1}} \; dx$
먼저, 식을 완전제곱식으로 바꾸면 $\frac{x}{\sqrt{x^{2} + x + 1}} = \frac{x}{\sqrt{(x + \frac{1}{2})^{2} + \frac{3}{4}}}$이다. 이제, $x + \frac{1}{2} = u$라고 두면 다음과 같이 적분할 수 있다.
$$\begin{align*} \int \frac{x}{\sqrt{x^{2} + x + 1}} \; dx &= \int \frac{x}{\sqrt{(x + \frac{1}{2})^{2} + \frac{3}{4}}} \; dx \\ &= \int \frac{u - \frac{1}{2}}{\sqrt{u^{2} +\frac{3}{4}}} \; du \\ &= \int \left( \frac{u}{\sqrt{u^{2} + \frac{3}{4}}} - \frac{1}{2} \cdot \frac{1}{\sqrt{u^{2} + \frac{3}{4}}} \right) \; du\end{align*}$$
1). $\int \frac{u}{\sqrt{u^{2} + \frac{3}{4}}} \; du$
먼저, $u^{2} +\frac{3}{4} = v$라고 두면 $2u du = dv \rightarrow du = \frac{1}{2u} dv$이다.
$$\begin{align*} \int \frac{u}{\sqrt{u^{2} + \frac{3}{4}}} \; du &= \int \frac{u}{\sqrt{v}} \cdot \frac{1}{2u} \; dv \\ &= \frac{1}{2} \int v^{-\frac{1}{2}} \; dv \\ &= \sqrt{v} + C_{1} \\ &= \sqrt{u^{2} + \frac{3}{4}} + C_{1} \\ &= \sqrt{x^{2} + x + 1} + C_{1} \end{align*}$$
2). $\int \frac{1}{\sqrt{u^{2} + \frac{3}{4}}} \; du$
먼저, $u = \frac{\sqrt{3}}{2} \tan(\theta)$라고 두면 $du = \frac{\sqrt{3}}{2} \sec^{2}(\theta) d\theta$이다.
$$\begin{align*} \int \frac{1}{\sqrt{u^{2} + \frac{3}{4}}} \; du &= \int \frac{1}{\sqrt{\frac{3}{4}\tan^{2}(\theta) + \frac{3}{4}}} \cdot \frac{\sqrt{3}}{2}\sec^{2}(\theta) \; d\theta \\ &= \int \sec(\theta) \; d\theta \\ &= \ln |\sec(\theta) + \tan(\theta)| + C_{2} \\ &= \ln \left| \frac{\sqrt{4u^{2} + 3} + 2u}{\sqrt{3}} \right| + C_{2} \\ &= \ln \left| \frac{2\left( \sqrt{x^{2} + x + 1} + (x + \frac{1}{2}) \right)}{\sqrt{3}} \right| + C_{2} \end{align*}$$
1)과 2)의 적분결과에 의해 기존 적분식은 다음과 같이 풀 수 있다.
$$\begin{align*} \int \frac{x}{\sqrt{x^{2} + x + 1}} \; dx &= \int \frac{x}{\sqrt{(x + \frac{1}{2})^{2} + \frac{3}{4}}} \; dx \\ &= \int \frac{u - \frac{1}{2}}{\sqrt{u^{2} +\frac{3}{4}}} \; du \\ &= \int \left( \frac{u}{\sqrt{u^{2} + \frac{3}{4}}} - \frac{1}{2} \cdot \frac{1}{\sqrt{u^{2} + \frac{3}{4}}} \right) \; du \\ &= \sqrt{x^{2} + x + 1} + \ln \left| \frac{2}{\sqrt{3}}\left( \sqrt{x^{2} + x + 1} + (x + \frac{1}{2}) \right) \right| + C \end{align*}$$
(b). $\int \frac{x^{2}}{(3 + 4x - 4x^{2})^{\frac{3}{2}}} \; dx$
먼저, 식을 완전제곱식으로 바꾸면 $\frac{x^{2}}{(3 + 4x - 4x^{2})^{\frac{3}{2}}} = \frac{x^{2}}{(4 - (2x - 1)^{2})^{\frac{3}{2}}}$이다. 이제, $2x - 1 = u$라고 두면 다음과 같이 적분할 수 있다.
$$\begin{align*} \int \frac{x^{2}}{(3 + 4x - 4x^{2})^{\frac{3}{2}}} \; dx &= \int \frac{x^{2}}{(4 - (2x - 1)^{2})^{\frac{3}{2}}} \; dx \\ &= \int \frac{\frac{1}{4}(u + 1)^{2}}{(4 - u^{2})^{\frac{3}{2}}} \; du \\ &= \frac{1}{4} \int \frac{u^{2} + 2u + 1}{(4 - u^{2})^{\frac{3}{2}}} \; du \\ &= \frac{1}{4} \int \left( \frac{u^{2}}{(4 - u^{2})^{\frac{3}{2}}} + 2\frac{u}{(4 - u^{2})^{\frac{3}{2}}} + \frac{1}{(4 - u^{2})^{\frac{3}{2}}} \right) \; du \end{align*}$$
1). $\int \frac{u^{2}}{(4 - u^{2})^{\frac{3}{2}}} \; du$
$u = 2\sin(\theta)$라고 두면 $du = 2\cos(\theta) d\theta$이다.
$$\begin{align*} \int \frac{u^{2}}{(4 - u^{2})^{\frac{3}{2}}} \; du \\ &= \int \frac{4\sin^{2}(\theta)}{(4 - 4\sin^{2}(\theta))^{\frac{3}{2}}} \cdot (2\cos(\theta)) \; d\theta \\ &= \int \frac{\sin^{2}(\theta)}{\cos^{2}(\theta)} \; d\theta \\ &= \int \tan^{2}(\theta) \; d\theta \\ &= \int (\sec^{2}(\theta) - 1) \; d\theta \\ &= \tan(\theta) - \theta + C_{1} \\ &= \frac{u}{\sqrt{4 - u^{2}}} - \arcsin(\frac{u}{2}) + C_{1} \\ &= \frac{2x - 1}{\sqrt{3 + 4x - 4x^{2}}} - \arcsin(\frac{1}{2}(2x - 1)) + C_{1} \end{align*}$$
2). $\int \frac{u}{(4 - u^{2})^{\frac{3}{2}}} \; du$
$4 - u^{2} = v$라고 두면 $-2u du = dv \rightarrow du = -\frac{1}{2u} dv$이다.
$$\begin{align*} \int \frac{u}{(4 - u^{2})^{\frac{3}{2}}} \; du &= \int \frac{u}{v^{\frac{3}{2}}} \cdot \left( -\frac{1}{2u} \right) \; dv \\ &= -\frac{1}{2} \int v^{-\frac{3}{2}} \; dv \\ &= -\frac{1}{2} \cdot \frac{1}{1 - \frac{3}{2}} v^{-\frac{1}{2}} + C_{2} \\ &= v^{-\frac{1}{2}} + C_{2} \\ &= \frac{1}{\sqrt{4 - u^{2}}} + C_{2} \\ &= \frac{1}{\sqrt{3 + 4x - x^{2}}} + C_{2} \end{align*}$$
3). $\int \frac{1}{(4 - u^{2})^{\frac{3}{2}}} \; du$
$u = 2\sin(\theta)$라고 두면 $du = 2\cos(\theta) d\theta$이다.
$$\begin{align*} \int \frac{1}{(4 - u^{2})^{\frac{3}{2}}} \; du \\ &= \int \frac{1}{(4 - 4\sin^{2}(\theta))^{\frac{3}{2}}} \cdot (2\cos(\theta)) \; d\theta \\ &= \frac{1}{4} \int \frac{1}{\cos^{2}(\theta)} \; d\theta \\ &= \frac{1}{4} \int \sec^{2}(\theta) \; d\theta \\ &= \frac{1}{4} \tan(\theta) + C_{3} \\ &= \frac{1}{4} \frac{u}{\sqrt{4 - u^{2}}} + C_{3} \\ &= \frac{2x - 1}{4\sqrt{3 + 4x - 4x^{2}}} + C_{3} \end{align*}$$
1), 2), 3)의 적분결과에 의해 기존 적분식은 다음과 같이 풀 수 있다.
$$\begin{align*} \int \frac{x^{2}}{(3 + 4x - 4x^{2})^{\frac{3}{2}}} \; dx &= \frac{1}{4} \int \left( \frac{u^{2}}{(4 - u^{2})^{\frac{3}{2}}} + 2\frac{u}{(4 - u^{2})^{\frac{3}{2}}} + \frac{1}{(4 - u^{2})^{\frac{3}{2}}} \right) \; du \\ &= \frac{1}{4} \left( \frac{2x - 1}{\sqrt{3 + 4x - 4x^{2}}} - \arcsin(\frac{1}{2}(2x - 1)) + \frac{2}{\sqrt{3 + 4x - x^{2}}} + \frac{2x - 1}{4\sqrt{3 + 4x - 4x^{2}}} \right) + C \end{align*}$$
(c). $\int \sqrt{x^{2} + 2x} \; dx$
먼저, 식을 완전제곱식으로 바꾸면 $\sqrt{x^{2} + 2x} = \sqrt{(x^{2} + 2x + 1) - 1} = \sqrt{(x + 1)^{2} - 1}$이다. 이제, $x + 1 = \sec(\theta)$라고 두면 다음과 같이 적분할 수 있다.
$$\begin{align*} \int \sqrt{x^{2} + 2x} \; dx &= \int \sqrt{(x + 1)^{2} - 1} \; dx \\ &= \int \sqrt{\sec^{2}(\theta) - 1} \cdot (\sec(\theta)\tan(\theta)) \; d\theta \\ &= \int \sec(\theta)\tan^{2}(\theta) \; d\theta \end{align*}$$
이제, $f = \tan(\theta), g^{'} = \sec(\theta)\tan(\theta)$라고 하면 $f^{'} = \sec^{2}(\theta), g = \sec(\theta)$이므로 부분적분을 적용하여 다음과 같이 적분할 수 있다.
$$\begin{align*} \int \sqrt{x^{2} + 2x} \; dx &= \int \sec(\theta)\tan^{2}(\theta) \; d\theta \\ &= \tan(\theta)\sec(\theta) - \int \sec^{3}(\theta) \; d\theta \\ &= \tan(\theta)\sec(\theta) - \int \sec(\theta) (1 + \tan^{2}(\theta)) \; d\theta \\ &= \tan(\theta)\sec(\theta) - \ln |\sec(\theta) + \tan(\theta)| - \int \sec(\theta)\tan^{2}(\theta) \; d\theta \\ \Rightarrow& \int \sqrt{x^{2} + 2x} \; dx = \int \sec(\theta)\tan^{2}(\theta) \; d\theta \\ &= \frac{1}{2} \left( \tan(\theta)\sec(\theta) - \frac{1}{2}\ln |\sec(\theta) + \tan(\theta)| \right) + C \\ &= \frac{1}{2} \left( \sqrt{x^{2} + 2x} - \ln \left| x + 1 + \frac{\sqrt{x^{2} + 2x}}{x + 1} \right| \right) + C \end{align*}$$
(d). $\int \frac{x^{2} + 1}{(x^{2} - 2x + 2)^{2}} \; dx$
먼저, 식을 완전제곱식으로 바꾸면 $\frac{x^{2} + 1}{(x^{2} - 2x + 2)^{2}} = \frac{x^{2} + 1}{((x - 1)^{2} + 1)^{2}}$이다. 이제, $x - 1 = u$라고 두면 다음과 같이 적분할 수 있다.
$$\begin{align*} \int \frac{x^{2} + 1}{(x^{2} - 2x + 2)^{2}} \; dx &= \int \frac{x^{2} + 1}{((x - 1)^{2} + 1)^{2}} \; dx \\ &= \int \frac{(u + 1)^{2} + 1}{(u^{2} + 1)^{2}} \; du \\ &= \int \frac{u^{2} + 2u + 2}{(u^{2} + 1)^{2}} \; du \\ &= \int \frac{u^{2} + 2u + 2}{(u^{2} + 1)^{2}} \; du \\ &= \int \left( \frac{u^{2}}{(u^{2} + 1)^{2}} + \frac{2u}{(u^{2} + 1)^{2}} + \frac{2}{(u^{2} + 1)^{2}} \right) \; du \end{align*}$$
1). $\int \frac{u^{2}}{(u^{2} + 1)^{2}} \; du$
$u = \tan(\theta)$라고 하면 $du = \sec^{2}(\theta) d\theta$이다.
$$\begin{align*} \int \frac{u^{2}}{(u^{2} + 1)^{2}} \; du &= \int \frac{\tan^{2}(\theta)}{(\tan^{2}(\theta) + 1)^{2}} \cdot \sec^{2}(\theta) \; d\theta \\ &= \int \frac{\tan^{2}(\theta}{\sec^{2}(\theta)} \; d\theta \\ &= \int \sin^{2}(\theta) \; d\theta \\ &= \int \frac{1 - \cos(2\theta)}{2} \; d\theta \\ &= \frac{1}{2} \left( \theta - \frac{1}{2}\sin(2\theta) \right) + C \\ &= \frac{1}{2} \left( \theta - \sin(\theta)\cos(\theta) \right) + C \\ &= \frac{1}{2} \left( \arctan(u) - \frac{u}{u^{2} + 1} \right) + C \\ &= \frac{1}{2} \left( \arctan(x - 1) - \frac{x - 1}{x^{2} - 2x + 2} \right) + C \end{align*}$$
2). $\int \frac{u}{(u^{2} + 1)^{2}} \; du$
$u^{2} + 1= v$라고 하면 $2u du = dv \rightarrow du = \frac{1}{2u} dv$이다.
$$\begin{align*} \int \frac{u}{(u^{2} + 1)^{2}} \; du &= \int \frac{u}{v^{2}} \cdot \frac{1}{2u} \; dv \\ &= \frac{1}{2} \int \frac{1}{v^{2}} \; dv \\ &= \frac{1}{2} \left( -\frac{1}{v} \right) + C_{2} \\ &= \frac{1}{2} \left( -\frac{1}{u^{2} + 1} \right) + C_{2} \\ &= \frac{1}{2} \left( -\frac{1}{x^{2} - 2x + 2} \right) + C_{2} \end{align*}$$
3). $\int \frac{1}{(u^{2} + 1)^{2}} \; du$
$u = \tan(\theta)$라고 하면 $du = \sec^{2}(\theta) \; d\theta$이다.
$$\begin{align*} \int \frac{1}{(u^{2} + 1)^{2}} \; du &= \int \frac{1}{(\tan^{2}(\theta) + 1)^{2}} \cdot \sec^{2}(\theta) \; d\theta \\ &= \int \frac{1}{\sec^{2}(\theta)} \; d\theta \\ &= \int \cos^{2}(\theta) \; d\theta \\ &= \int \frac{1 + \cos(2\theta)}{2} \; d\theta \\ &= \frac{1}{2} \left( \theta + \frac{1}{2}\sin(2\theta) \right) + C_{3} \\ &= \frac{1}{2} \left( \theta + \sin(\theta)\cos(\theta) \right) + C_{3} \\ &= \frac{1}{2} \left( \arctan(u) + \frac{u}{u^{2} + 1} \right) + C_{3} \\ &= \frac{1}{2} \left( \arctan(x - 1) + \frac{x - 1}{x^{2} - 2x + 2} \right) + C_{3} \end{align*}$$
1), 2), 3)의 적분결과에 의해 기존 적분식은 다음과 같이 풀 수 있다.
$$\begin{align*} \int \frac{x^{2} + 1}{(x^{2} - 2x + 2)^{2}} \; dx &= \int \left( \frac{u^{2}}{(u^{2} + 1)^{2}} + \frac{2u}{(u^{2} + 1)^{2}} + \frac{2}{(u^{2} + 1)^{2}} \right) \; du \\ &= \frac{1}{2} \left( \arctan(x - 1) - \frac{x - 1}{x^{2} - 2x + 2} \right) -\frac{1}{x^{2} - 2x + 2} + \arctan(x - 1) + \frac{x - 1}{x^{2} - 2x + 2} + C \\ &= \frac{3}{2} \arctan(x - 1) + \frac{x - \frac{3}{2}}{2(x^{2} - 2x + 2)} + C \end{align*}$$
(e). $\int x\sqrt{1 - x^{4}} \; dx$
$x = \sqrt{\sin(\theta)}$라고 하면 $dx = \frac{\cos(\theta)}{2\sqrt{\sin(\theta)}} \; d\theta$이다.
$$\begin{align*} \int x\sqrt{1 - x^{4}} \; dx &= \int \sqrt{\sin(\theta)} \sqrt{1 - \sin^{2}(\theta)} \cdot \frac{\cos(\theta)}{2\sqrt{\sin(\theta)}} \; d\theta \\ &= \frac{1}{2} \int \cos^{2}(\theta) \; d\theta \\ &= \frac{1}{2} \int \frac{1 + \cos(2\theta)}{2} \; d\theta \\ &= \frac{1}{4} \int \left( \theta + \frac{1}{2}\sin(2\theta) \right) \; d\theta \\ &= \frac{1}{4} \left( \theta + \sin(\theta)\cos(\theta) \right) + C \\ &= \frac{1}{4} \left( \arcsin(x^{2}) - x^{2}\sqrt{1 - x^{2}} \right) \end{align*}$$
연습문제5. 두 적분을 증명하라.
(a). 삼각함수 치환법을 통해 $\int \frac{1}{\sqrt{x^{2} + a^{2}}} \; dx = \ln (x + \sqrt{x^{2} + a^{2}}) + C$임을 증명하라.
(b). 쌍곡함수 치환법을 통해 $\int \frac{1}{\sqrt{x^{2} + a^{2}}} \; dx = \arcsinh \left( \frac{a}{x} \right) + C$임을 증명하라.
(a). 삼각함수 치환법을 통해 $\int \frac{1}{\sqrt{x^{2} + a^{2}}} \; dx = \ln (x + \sqrt{x^{2} + a^{2}}) + C$임을 증명하라.
$x = a\tan(\theta)$라고 하면 $dx = a\sec^{2}(\theta) \; d\theta$이다.
$$\begin{align*} \int \frac{1}{\sqrt{x^{2} + a^{2}}} \; dx &= \int \frac{1}{\sqrt{a^{2}\tan^{2}(\theta) + a^{2}}} \cdot (a\sec^{2}(\theta)) \; d\theta \\ &= \int \sec(\theta) \; d\theta \\ &= \ln (\sec(\theta) + \tan(\theta)) + C \\ &= \ln (x + \sqrt{x^{2} + a^{2}}) + C \end{align*}$$
(b). 쌍곡함수 치환법을 통해 $\int \frac{1}{\sqrt{x^{2} + a^{2}}} \; dx = \arcsinh \left( \frac{a}{x} \right) + C$임을 증명하라.
$x = a\sinh(\theta)$라고 하면 $dx = a\cosh(\theta) \; d\theta$이다.
$$\begin{align*} \int \frac{1}{\sqrt{x^{2} + a^{2}}} \; dx &= \int \frac{1}{\sqrt{a^{2}\sinh^{2}(\theta) + a^{2}}} \cdot (a\cosh(\theta)) \; d\theta \\ &= \int 1 \; d\theta \\ &= \theta + C \\ &= \arcsinh \left( \frac{x}{a} \right) + C \end{align*}$$
연습문제6. 주어진 영역의 넓이를 구하여라. 여기서, 부채꼴의 반지름은 $r$이고 각은 $\theta$이다.

주어진 영역의 넓이는 $\triangle OPQ$와 호 $PQR$의 넓이의 합이다.
1). $\triangle OPQ$의 넓이를 A라고 할 때 삼각형이므로 $A = \frac{(r\cos(theta) \cdot (r\sin(\theta))}{2} = \frac{r^{2}}{2}(\sin(\theta)\cos(\theta))$이다.
2). 호 $PQR$의 넓이를 $B$라고 할 때 해당 영역은 원 $x^{2} + y^{2} = r^{2}$에서 구간 $[r\cos(\theta), r]$에서의 밑넓이와 동일하므로 다음과 같이 적분할 수 있다.
$$\begin{align*} B &= \int_{r\cos(\theta)}^{r} \sqrt{r^{2} - x^{2}} \; dx \end{align*}$$
이를 위해 $x = r\cos(\phi)$라고 하면 $dx = -r\sin(\phi) d\phi$이다.
$$\begin{align*} \int \sqrt{r^{2} - x^{2}} \; dx &= \int \sqrt{r^{2} - r^{2}\cos^{2}(\phi)} \cdot (-r\sin(\phi)) \; d\theta \\ &= \int -r^{2}\sin^{2}(\phi) \; d\phi \\ &= -r^{2} \int \frac{1 - \cos(2\phi)}{2} \; d\phi \\ &= -\frac{r^{2}}{2} \left( \phi - \frac{1}{2}\sin(2\phi) \right) + C \\ &= -\frac{r^{2}}{2} \left(\phi - \sin(\phi)\cos(\phi) \right) + C \\ &= -\frac{r^{2}}{2} \left( \arccos(\frac{x}{r}) - \frac{x\sqrt{r^{2} - x^{2}}}{r^{2}} \right) + C \\ &= \frac{r^{2}}{2} \left( \frac{x\sqrt{r^{2} - x^{2}}}{r^{2}} - \arccos(\frac{x}{r}) \right)\end{align*}$$
따라서 다음과 같이 정적분을 계산할 수 있다.
$$\begin{align*} B &= \int_{r\cos(\theta)}^{r} \sqrt{r^{2} - x^{2}} \; dx \\ &= \frac{r^{2}}{2} \left[ \frac{x\sqrt{r^{2} - x^{2}}}{r^{2}} - \arccos(\frac{r}{x}) \right]_{r\cos(\theta)}^{r} \\ &= \frac{r^{2}}{2} \left[ \left( 0 - \arccos(1) \right) - \left( \frac{r^{2}\cos(\theta)\sin(\theta)}{r^{2}} - \arccos(\cos(\theta)) \right) \right] \\ &= -\frac{r^{2}}{2} \sin(\theta)\cos(\theta) + \frac{r^{2}}{2}\theta \end{align*}$$
이제, 1), 2)의 적분결과를 합치면 된다.
$$A + B = \left( \frac{r^{2}}{2} \sin(\theta)\cos(\theta) \right) + \left( -\frac{r^{2}}{2}\sin(\theta)\cos(\theta) + \frac{r^{2}}{2} \theta \right) = \frac{r^{2}}{2} \theta $$
참고자료 및 그림출처
Calculus(J. Stewart)
'수학 > 미적분학' 카테고리의 다른 글
| 미적분학 - 적분 전략 (0) | 2022.03.24 |
|---|---|
| 미적분학 - 부분분수를 활용한 유리함수 적분 (0) | 2022.03.22 |
| 미적분학 - 복잡한 삼각함수 적분 (0) | 2022.03.16 |
| 미적분학 - 부분적분 (0) | 2022.03.14 |
| 미적분학 - 함수의 평균 (0) | 2022.03.12 |
